M10 Der Logarithmus: Unterschied zwischen den Versionen
(Die Seite wurde neu angelegt: „Die Gleichung <math>2^x = 4</math> ist ganz leicht zu lösen. Man erhält <math> x = 2</math>. Dies geht immer gut, wenn der Wert auf der rechten Seite eine Po…“) |
|||
| (26 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 5: | Zeile 5: | ||
Doch was macht man, wenn die Gleichung <math>2^x = 5 </math> lautet? | Doch was macht man, wenn die Gleichung <math>2^x = 5 </math> lautet? | ||
| + | |||
| + | Man hatte schon einmal ein ähnliches Problem. Die Gleichung <math>x^2 = 4</math> hat die Lösungen <math>x_1 = -2</math> und <math>x_2=2</math>. Für die Gleichung <math>x^2 = 5</math> hat man dann neue Zahlen eingeführt, die Wurzeln, und die Gleichung hatte die Lösungen <math>x_1 = -\sqrt 5, x_2 = \sqrt 5</math>. | ||
| + | |||
| + | Für die Gleichung <math>2^x = 5 </math> muss man, um eine Lösung zu haben, neue Zahlen einführen, die Logarithmen bzw. den Logarithmus. | ||
<center>{{#ev:youtube |oDOXeO9fAg4|350}}</center> | <center>{{#ev:youtube |oDOXeO9fAg4|350}}</center> | ||
| Zeile 11: | Zeile 15: | ||
{{Merksatz|MERK=Die Gleichung <math>a^x = p</math> mit a <math>\in</math> R<sup>+</sup> und p > 0 hat die Lösung <math>x = log_a (p)</math>. | {{Merksatz|MERK=Die Gleichung <math>a^x = p</math> mit a <math>\in</math> R<sup>+</sup> und p > 0 hat die Lösung <math>x = log_a (p)</math>. | ||
| − | Man spricht für <math>x = log_a (p)</math>: "'''x ist der Logarithmus von p zur Basis a'''" }} | + | Man spricht für <math>x = log_a (p)</math>: "'''x ist der Logarithmus von p zur Basis a'''" |
| + | |||
| + | <center>[[Datei:Log.jpg|500px]]</center>}} | ||
| Zeile 18: | Zeile 24: | ||
<math>10^x = 5</math> hat die Lösung <math> x = log_{10}(5)</math><br> | <math>10^x = 5</math> hat die Lösung <math> x = log_{10}(5)</math><br> | ||
<math>2^x = 19 </math> hat die Lösung <math>x = log_2[18)</math> | <math>2^x = 19 </math> hat die Lösung <math>x = log_2[18)</math> | ||
| + | |||
| + | <center>{{#ev:youtube |iuG7isoQjGc|350}}</center> | ||
| + | |||
| + | {{Aufgaben-blau|1|2=1. Schreibe den Exponenten als Logarithmus<br> | ||
| + | a) <math>2^3 = 8</math><br> | ||
| + | b) <math>5^4 = 625</math><br> | ||
| + | c) <math>2^{-3} = \frac{1}{8}</math><br> | ||
| + | d) <math>7^0 = 1</math><br> | ||
| + | e) <math>10^{-2}=0,01</math><br> | ||
| + | f) <math>100^{\frac{1}{2}}=10</math> | ||
| + | |||
| + | 2. Schreibe die Logarithmusgleichung als Exponentialgleichung<br> | ||
| + | a) <math>log_3(9) = 2</math><br> | ||
| + | b) <math>log_4(16) = 2</math><br> | ||
| + | c) <math>log_4(\frac{1}{16}) = -2</math><br> | ||
| + | d) <math>log_8(0,125) = -1</math> <br> | ||
| + | e) <math>log_3(\frac{1}{81})= -4</math> }} | ||
| + | |||
| + | {{Lösung versteckt|1= 1a) <math>3 = log_2(8)</math><br> | ||
| + | b) <math>4 = log_5(625)</math><br> | ||
| + | c) <math>-3 = log_2(\frac{1}{8})</math><br> | ||
| + | d) <math>0 = log_7(1)</math><br> | ||
| + | e) <math>-2 = log_{10}(0,1)</math><br> | ||
| + | f) <math>\frac{1}{2}=log_{100}(10)</math> | ||
| + | |||
| + | 2a) <math>3^2 = 9</math><br> | ||
| + | b) <math>4^2 = 16</math><br> | ||
| + | c) <math>4^{-2}=\frac{1}{16}</math><br> | ||
| + | d) <math>8^{-1}=0,125</math><br> | ||
| + | e) <math>3^{-4}=\frac{1}{81}</math> }} | ||
| + | |||
| + | {{Merksatz|MERK=Es ist <math>log_a(a) = 1</math> | ||
| + | |||
| + | <math>log_a(a^r) = r</math> | ||
| + | |||
| + | <math>log_a(1) = 0</math>}} | ||
| + | |||
| + | {{Aufgaben-blau|2|2=1. Beerechne ohne TR<br> | ||
| + | a) log<sub>2</sub>32<br> | ||
| + | b) log<sub>2</sub>1024<br> | ||
| + | c) log<sub>3</sub>243<br> | ||
| + | d) log<sub>7</sub>7<br> | ||
| + | e) log<sub>10</sub>10000<br> | ||
| + | f) log<sub>10</sub>1<br> | ||
| + | g) log<sub>2</sub>0,5<br> | ||
| + | h) log<sub>2</sub><math>\frac{1}{8}</math><br> | ||
| + | i) log<sub>2</sub>0,25<br> | ||
| + | k) log<sub>5</sub>0,2<br> | ||
| + | l) log<sub>5</sub>0,04<br> | ||
| + | m) log<sub>5</sub>0,008<br> | ||
| + | n) log<sub>10</sub>0,1<br> | ||
| + | o) log<sub>0,1</sub>10<br> | ||
| + | p) log<sub>0,1</sub>1000<br> | ||
| + | q) log<sub>0,1</sub>0,01<br> | ||
| + | r) log<sub>0,1</sub>1<br> | ||
| + | s) log<sub>0,5</sub><math>\frac{1}{4}</math><br> | ||
| + | t) log<sub>0,5</sub><math>\frac{1}{2}</math><br> | ||
| + | u) log<sub>0,5</sub>2<br> | ||
| + | v) log<sub>2,5</sub>6,25<br> | ||
| + | w) log<sub>2,5</sub>1<br> | ||
| + | x) log<sub>2,5</sub>0,4<br> | ||
| + | y) log<sub>2,5</sub>0,16 | ||
| + | |||
| + | 2. Berechne ohne TR<br> | ||
| + | a) <math>log_7(\sqrt 7)</math><br> | ||
| + | b) <math>log_4(2)</math><br> | ||
| + | c) <math>log_2(\sqrt[3]2)</math><br> | ||
| + | d) <math>log_{27}(3)</math><br> | ||
| + | e) <math>log_{32}(2)</math><br> | ||
| + | f) <math>log_5(\sqrt[3]{25})</math><br> | ||
| + | g) <math>log_3(\sqrt{27})</math><br> | ||
| + | h) <math>log_2(2\sqrt 2)</math><br> | ||
| + | i) <math>log_2(8\sqrt 8)</math><br> | ||
| + | k) <math>log_{100}(10)</math><br> | ||
| + | l) <math>log_{100}(1000)</math><br> | ||
| + | m) <math>log_{100}(0,001)</math><br> | ||
| + | n) <math>log_{\sqrt 3}(3)</math><br> | ||
| + | o) <math>log_{\sqrt 6}(\frac{1}{6})</math><br> | ||
| + | p) <math>log_{\sqrt 2}(2^{-3})</math><br> | ||
| + | q) <math>log_{\sqrt 7}(1)</math><br> | ||
| + | |||
| + | |||
| + | Tipp: Stelle die passende Exponentialgleichung auf!<br> | ||
| + | Für log<sub>2</sub>(32) lautet die Exponentialgleichung <math>2^x = 32</math>, also x = 5}} | ||
| + | |||
| + | {{Lösung versteckt|1=Stelle eventuell die passende Exponentialgleichung auf!<br> | ||
| + | Für log<sub>2</sub>(32) lautet die Exponentialgleichung <math>2^x = 32</math>, also x = 5 | ||
| + | |||
| + | 1a) 5; b) 10; c) 5; d) 1; e) 4; f) 0; g) -1; h) -3; i) -2; k) -1; l) -2; m) -3<br> | ||
| + | n) -1; o) -1; p) -3; q) 2; r) 0; s) 2; t) 1; u) -1; v) 2; w) 0; x) -1; y) -2 | ||
| + | |||
| + | 2a) 0,5; b) 0,5; c) <math>\frac{1}{3}</math>; d) <math>\frac{1}{3}</math>; e) <math>\frac{1}{5}</math>; f) <math>\frac{2}{3}</math>; g) <math>\frac{3}{2}</math>; h) <math>\frac{3}{2}</math>;;<br> | ||
| + | i) <math>\frac{9}{2}</math>; k) 0,5; l) <math>\frac{3}{2}</math>; m) <math>-\frac{3}{2}</math>; o) 2; p) -6; q) 0 }} | ||
| + | |||
| + | {{Aufgaben-blau|3|2=Logarithmiere<br> | ||
| + | a) <math>log_a(a^3)</math><br> | ||
| + | b) <math>log_a(a^5)</math><br> | ||
| + | c) <math>log_a(1)</math><br> | ||
| + | d) <math>log_a(\frac{1}{a^2})</math><br> | ||
| + | e) <math>log_a(\frac{1}{a})</math><br> | ||
| + | f) <math>log_a(a)</math><br> | ||
| + | g) <math>log_a(\sqrt a)</math><br> | ||
| + | h) <math>log_a(\sqrt[5]{a})</math><br> | ||
| + | i) <math>log_a(\sqrt[4]{a^3})</math><br> | ||
| + | k) <math>log_a(\frac{1}{\sqrt a})</math><br> }} | ||
| + | |||
| + | {{Lösung versteckt|1=a) <math>log_a(a^3)= 3</math><br> | ||
| + | b) <math>log_a(a^5)= 5</math><br> | ||
| + | c) <math>log_a(1)= 0</math><br> | ||
| + | d) <math>log_a(\frac{1}{a^2})= -2</math><br> | ||
| + | e) <math>log_a(\frac{1}{a})= -1</math><br> | ||
| + | f) <math>log_a(a)=1</math><br> | ||
| + | g) <math>log_a(\sqrt a)=\frac{1}{2}</math><br> | ||
| + | h) <math>log_a(\sqrt[5]{a})=\frac{1}{5}</math><br> | ||
| + | i) <math>log_a(\sqrt[4]{a^3})=\frac{3}{4}</math><br> | ||
| + | k) <math>log_a(\frac{1}{\sqrt a})=-\frac{1}{2}</math><br> }} | ||
| + | |||
| + | <center>{{#ev:youtube |2vIZNqYHpos|350}}</center> | ||
| + | |||
| + | |||
| + | {{Merksatz|MERK=Rechengesetze des Logarithmus | ||
| + | |||
| + | Logarithmus eines Produkts: <math>log_a(p\cdot q) = log_a(p) + log_a(q)</math> | ||
| + | |||
| + | Logarithmus eines Quotienten: <math>log_a(\frac{p}{q})=log_a(p) - log_a(q)</math> | ||
| + | |||
| + | Logarithmus einer Potenz: <math>log_a(p^r) = r\cdot log_a(p)</math> }} | ||
| + | |||
| + | |||
| + | Zur Begründung der Rechenregeln:<br> | ||
| + | Man geht bei den Begründungen auf die Definition des Logarithmus zurück und macht dann entsprechende Umformungen bei den Exponentialgleichungen. Das Ergebnis erhält man, wenn man die Exponenten vergleicht. <br> | ||
| + | 1. <math>log_a(p\cdot q) = log_a(p) + log_a(q)</math> erhält man durch folgende Überlegung:<br> | ||
| + | <math>p = b^x</math> und <math>q = b^y</math>. Dann ist <math>p\cdot q = b^x \cdot b^y = b^{x+y}</math>, also <math>x + y = log_b(p\cdot q)</math>.<br> | ||
| + | Da <math>x = log_b (p)</math> und <math>y = log_b(q)</math> ist erhält man <math>log_b(p)+log_b(q)=x+y=log_b(p\cdot q)</math>. | ||
| + | |||
| + | 2. <math>log_a(\frac{p}{q}) = log_a(p) - log_a(q)</math> erhält man durch folgende Überlegung:<br> | ||
| + | <math>p = b^x</math> und <math>q = b^y</math>. Dann ist <math>p : q = b^x : b^y = b^{x-y}</math>, also <math>x - y = log_b(p : q)</math>.<br> | ||
| + | Da <math>x = log_b (p)</math> und <math>y = log_b(q)</math> ist erhält man <math>log_b(p)-log_b(q)=x-y=log_b(p: q)=\log_b(\frac{p}{q})</math>. | ||
| + | |||
| + | 3. Es ist <math>a^{log_a(p^r)} = p^r = (a^{log_a(p)})^r=a^{r\cdot log_a(p)}</math>. Zwei Potenzen mit gleicher Basis haben denselben Wert, wenn auch ihre Exponenten gleich sind, also <math>log_a(p^r) = r\cdot log_a(p)</math>. | ||
| + | |||
| + | <center>{{#ev:youtube |gKtM31rf_7s|350}}</center> | ||
| + | |||
| + | '''Beispiele:1. <math>log_3(9a^4)=log_3(9)+log_3(a^4)=log_3(3^2)-4\cdot log_3(a) = 2 + 4log_3(a)</math> | ||
| + | |||
| + | 2. <math>log_{10}(1000\cdot\sqrt[5]{a^2}=log_{10}(1000)+log_{10}(a^{\frac{2}{5}})=3 +\frac{2}{5}log_{10}(a)</math> | ||
| + | |||
| + | 3. <math>log_2 (6) log_2(48) = log_2(\frac{6}{48})=log_2(\frac{1}{8})=log_2(2^{-3})=-3</math> | ||
| + | |||
| + | {{Merke|1=Für <math>log_{10}</math> schreibt man <math>lg</math> | ||
| + | |||
| + | Für <math>log_e</math> schreibt man <math>ln</math>, wenn e die Eulersche Zahl e = 2, 718 281 828 459 045 235 360 287 ... ist. | ||
| + | |||
| + | Diese beiden Symbole findest du auch auf dem Taschenrechner.}} | ||
| + | |||
| + | 4. <math>lg(\sqrt {250})-lg(\sqrt 2)+0,5lg(8)=lg({\frac{\sqrt {250} \cdot \sqrt 8}{\sqrt 2}}= lg(\sqrt{1000} =\lg(10^{\frac{3}{2}})=\frac{3}{2}</math> | ||
| + | |||
| + | {{Aufgaben-blau|4|2=Für die Logarithmen zur Basis 2 hat man diese Tabelle: | ||
| + | [[Datei:Log zur Basis 2.jpg]] | ||
| + | |||
| + | Berechne die Logarithmen auf zwei verschiedene Arten: <br> | ||
| + | 1.) indem du zuerst das Argument des Logarithmus berechnest und dann logarithmierst.<br> | ||
| + | 2.) indem die Rechenregeln verwendest und die Logarithmen addierst, subtrahierst, .... | ||
| + | |||
| + | Beispiel: log<sub>2</sub>(4·8) berechnest du als <br> | ||
| + | 1.) log<sub>2</sub>(4·8)=log<sub>2</sub>(32)=5<br> | ||
| + | 2.) log<sub>2</sub>(4·8) = log<sub>2</sub>(4) + log<sub>2</sub>(8)= 2 + 3 = 5 | ||
| + | |||
| + | a) log<sub>2</sub>(4·16)<br> | ||
| + | b) log<sub>2</sub>(4·32)<br> | ||
| + | c) log<sub>2</sub>(8·32)<br> | ||
| + | d) log<sub>2</sub>(<math>\frac{32}{8}</math>)<br> | ||
| + | e) log<sub>2</sub>(<math>\frac{64}{4}</math>)<br> | ||
| + | f) log<sub>2</sub>(<math>\frac{128}{8}</math>)<br> | ||
| + | g) log<sub>2</sub>(<math>\frac{256}{32}</math>)<br> | ||
| + | h) log<sub>2</sub>(4<sup>3</sup>)<br> | ||
| + | i) log<sub>2</sub>(8<sup>5</sup>) }} | ||
| + | |||
| + | {{Lösung versteckt|1=a) 1.) log<sub>2</sub>(4·16)=log(64)=6<br> | ||
| + | 2.) log<sub>2</sub>(4·16)= log<sub>2</sub>(4) + log<sub>2</sub>(16) = 2 + 4 = 6 | ||
| + | |||
| + | b) 1.) log<sub>2</sub>(4·32)= log<sub>2</sub>(128) = 7<br> | ||
| + | 2.) log<sub>2</sub>(4·16) = log<sub>2</sub>(4) + log<sub>2</sub>(16) ? 2 + 4 = 6 | ||
| + | |||
| + | c) 1.) log<sub>2</sub>(8·32) = log<sub>2</sub>(256) = 8<br> | ||
| + | 2.) log<sub>2</sub>(8·32)= log<sub>2</sub>(8) + log<sub>2</sub>(32) = 3 + 5 = 8 | ||
| + | |||
| + | d) 1.) log<sub>2</sub>(<math>\frac{32}{8}</math>) = log<sub>2</sub>(4) = 2<br> | ||
| + | 2.) log<sub>2</sub>(<math>\frac{32}{8}</math>) = log<sub>2</sub>(32) - log<sub>2</sub>(8) = 5 - 3 = 2 | ||
| + | |||
| + | e) 1.) log<sub>2</sub>(<math>\frac{64}{4}</math>) = log<sub>2</sub>(16) = 4<br> | ||
| + | 2.) log<sub>2</sub>(<math>\frac{64}{4}</math>) = log<sub>2</sub>(64) - log<sub>2</sub>(4) = 6 - 2 = 4 | ||
| + | |||
| + | f) 1.) log<sub>2</sub>(<math>\frac{128}{8}</math>) = log<sub>2</sub>(16) = 4<br> | ||
| + | 2.) log<sub>2</sub>(<math>\frac{128}{8}</math>) = log<sub>2</sub>(128) - log<sub>2</sub>(8) = 7 - 3 = 4 | ||
| + | |||
| + | g) 1.) log<sub>2</sub>(<math>\frac{256}{32}</math>) = g) log<sub>2</sub>(8) = 3<br> | ||
| + | 2.) g) log<sub>2</sub>(<math>\frac{256}{32}</math>) = log<sub>2</sub>(256) - log<sub>2</sub>(32) = 8 - 5 = 3 | ||
| + | |||
| + | h) 1.) log<sub>2</sub>(4<sup>3</sup>) = log<sub>2</sub>(512) = 9<br> | ||
| + | 2.) log<sub>2</sub>(4<sup>3</sup>) = 3·log<sub>2</sub>(4) = 3·3 = 9 | ||
| + | |||
| + | i) 1.) log<sub>2</sub>(8<sup>5</sup>) = log<sub>2</sub>(32768) = 15<br> | ||
| + | 2.) log<sub>2</sub>(8<sup>5</sup>) = 5·log<sub>2</sub>(8) = 5·3 = 15 }} | ||
| + | |||
| + | |||
| + | {{Merke|1='''Nicht verwechseln!''' | ||
| + | |||
| + | <center>[[Datei:Potenzieren.jpg|300px]] </center> }} | ||
| + | |||
| + | |||
| + | {{Merksatz|MERK=<center>{{#ev:youtube |ptu_0F0hNd8|350}}</center> | ||
| + | |||
| + | '''Basiswechsel''': <math> log_a(p) = \frac{log_b(p)}{log_b(a)}</math> }} | ||
| + | |||
| + | Zur Begründung: <math>x = log_a(p)</math> ist Lösung der Gleichung <math>a^x = p</math>.<br> | ||
| + | Nun möchte man die Basis a durch die Basis b ersetzen. Dazu verwendet man, dass <math> a = b^{log_b(a)}</math> ist. <br> | ||
| + | Es ist dann <math>a^x = (b^{log_b(a)})^x = b^{x \cdot log_b(a)}</math> und die Gleichung lautet dann <math>b^{x \cdot log_b(a)}=p</math><br> | ||
| + | Diese Gleichung löst man nach dem Exponenten auf. Es ist <math>x \cdot log_b(a) = log_b(p)</math>, dividiert durch den Koeffizienten von x und erhält <math> x = \frac{log_b(p)}{log_b(a)}</math>.<br> | ||
| + | Damit hat man gezeigt, dass <math>log_a(p) = x = \frac{log_b(p)}{log_b(a)}</math> ist. | ||
| + | |||
| + | Beispiele: Auf den Taschenrechnern sind immer zwei Logarithmus-Tasten, meist eine Taste log oder lg für den Logarithmus zur Basis 10 und ln für den Logarithmus zur Basis e. <br> | ||
| + | <math>log_2(5) = \frac{lg(5)}{lg(2)}\approx 2,231928</math><br> | ||
| + | <math>log_7(2)=\frac{ln(2)}{ln(7)}\approx 0,359207</math> | ||
| + | |||
| + | {{Aufgaben-blau|5|2=Vervollständige die Tabellen mit Hilfe deines Taschenrechners:<br> | ||
| + | 1. [[Datei:Log zur basis 10.jpg]] | ||
| + | |||
| + | 2. Verwende die Formel für den Basiswechsel! <br> | ||
| + | [[Datei:Log zur Basis 2 2.jpg]] }} | ||
| + | |||
| + | {{Lösung versteckt|1=1. [[Datei:Log zur basis 10 2.jpg]] | ||
| + | |||
| + | 2. [[Datei:Log zur Basis 2 2a.jpg]] <br> | ||
| + | Ich habe diese Tabelle mit einer Tabellenkalkulation erstellt, dort gibt man auch die Basis ein, also berechnet man tatsächlich den gewünschen Logarithmus. Ihr sollt aber ja den Basiswechsel am TR üben. }} | ||
| + | |||
| + | {{Aufgaben-blau|6|2=Bearbeite die Aufgaben auf <br> | ||
| + | 1. [https://de.serlo.org/mathe/23768/aufgaben-zum-rechnen-mit-logarithmen dieser Seite]. <br> | ||
| + | 2. [http://www.mathe-trainer.de/Klasse10/Exponentialfunktion/Block5/Aufgaben.htm dieser Seite].<br> | ||
| + | 3. [https://mathe.aufgabenfuchs.de/potenz/logarithmus.shtml dieser Seite]. }} | ||
Aktuelle Version vom 21. November 2022, 08:37 Uhr
Die Gleichung 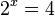 ist ganz leicht zu lösen. Man erhält
ist ganz leicht zu lösen. Man erhält 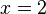 . Dies geht immer gut, wenn der Wert auf der rechten Seite eine Potenz der Basis ist, also
. Dies geht immer gut, wenn der Wert auf der rechten Seite eine Potenz der Basis ist, also
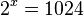 hat die Lösung
hat die Lösung 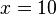 ,
,
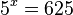 hat die Lösung
hat die Lösung 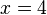 ,
,
 hat die Lösung
hat die Lösung 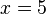 .
.
Doch was macht man, wenn die Gleichung  lautet?
lautet?
Man hatte schon einmal ein ähnliches Problem. Die Gleichung 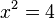 hat die Lösungen
hat die Lösungen 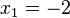 und
und 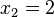 . Für die Gleichung
. Für die Gleichung 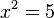 hat man dann neue Zahlen eingeführt, die Wurzeln, und die Gleichung hatte die Lösungen
hat man dann neue Zahlen eingeführt, die Wurzeln, und die Gleichung hatte die Lösungen 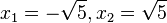 .
.
Für die Gleichung  muss man, um eine Lösung zu haben, neue Zahlen einführen, die Logarithmen bzw. den Logarithmus.
muss man, um eine Lösung zu haben, neue Zahlen einführen, die Logarithmen bzw. den Logarithmus.
|
Merke:
Die Gleichung Man spricht für  |
Beispiele: 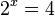 hat die Lösung
hat die Lösung 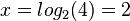
 hat die Lösung
hat die Lösung 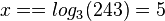
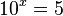 hat die Lösung
hat die Lösung 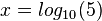
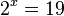 hat die Lösung
hat die Lösung 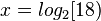
|
Merke:
Es ist
|
|
Merke:
Rechengesetze des Logarithmus Logarithmus eines Produkts: Logarithmus eines Quotienten: Logarithmus einer Potenz: |
Zur Begründung der Rechenregeln:
Man geht bei den Begründungen auf die Definition des Logarithmus zurück und macht dann entsprechende Umformungen bei den Exponentialgleichungen. Das Ergebnis erhält man, wenn man die Exponenten vergleicht.
1. 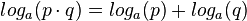 erhält man durch folgende Überlegung:
erhält man durch folgende Überlegung:
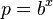 und
und 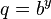 . Dann ist
. Dann ist 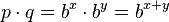 , also
, also 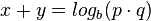 .
.
Da  und
und 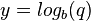 ist erhält man
ist erhält man  .
.
2.  erhält man durch folgende Überlegung:
erhält man durch folgende Überlegung:
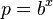 und
und 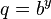 . Dann ist
. Dann ist 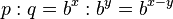 , also
, also  .
.
Da  und
und 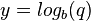 ist erhält man
ist erhält man 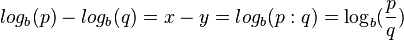 .
.
3. Es ist 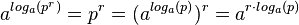 . Zwei Potenzen mit gleicher Basis haben denselben Wert, wenn auch ihre Exponenten gleich sind, also
. Zwei Potenzen mit gleicher Basis haben denselben Wert, wenn auch ihre Exponenten gleich sind, also 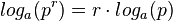 .
.
Beispiele:1. 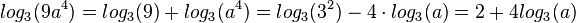
2. ![log_{10}(1000\cdot\sqrt[5]{a^2}=log_{10}(1000)+log_{10}(a^{\frac{2}{5}})=3 +\frac{2}{5}log_{10}(a)](/images/math/b/0/4/b04b343917aa8efa40b64fd674cc11fb.png)
3. 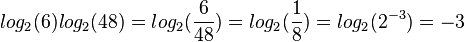
|
Für Für Diese beiden Symbole findest du auch auf dem Taschenrechner. |
4. 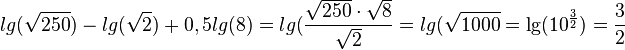
|
Nicht verwechseln! 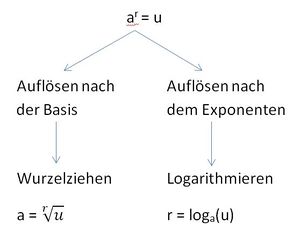 |
|
Merke:
Basiswechsel: |
Zur Begründung:  ist Lösung der Gleichung
ist Lösung der Gleichung 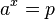 .
.
Nun möchte man die Basis a durch die Basis b ersetzen. Dazu verwendet man, dass 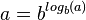 ist.
ist.
Es ist dann 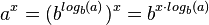 und die Gleichung lautet dann
und die Gleichung lautet dann 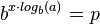
Diese Gleichung löst man nach dem Exponenten auf. Es ist 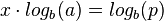 , dividiert durch den Koeffizienten von x und erhält
, dividiert durch den Koeffizienten von x und erhält  .
.
Damit hat man gezeigt, dass 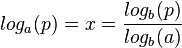 ist.
ist.
Beispiele: Auf den Taschenrechnern sind immer zwei Logarithmus-Tasten, meist eine Taste log oder lg für den Logarithmus zur Basis 10 und ln für den Logarithmus zur Basis e.
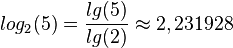
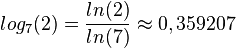

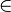 R+ und p > 0 hat die Lösung
R+ und p > 0 hat die Lösung 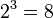
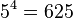
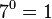
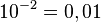

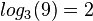
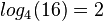



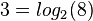

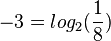
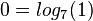
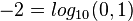
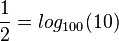

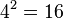
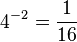
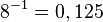

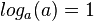
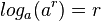
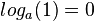



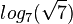
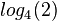
![log_2(\sqrt[3]2)](/images/math/8/9/a/89ad42e90d43beb69625ac066902118b.png)
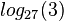
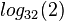
![log_5(\sqrt[3]{25})](/images/math/4/5/e/45e91e4c37d394a4d33f0158fb5cb2c3.png)
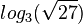
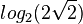
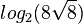
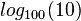
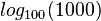
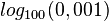
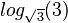
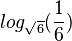
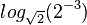

 , also x = 5
, also x = 5
 ; d)
; d)  ; f)
; f)  ; g)
; g)  ; h)
; h)  ; k) 0,5; l)
; k) 0,5; l) 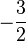 ; o) 2; p) -6; q) 0
; o) 2; p) -6; q) 0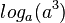
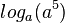
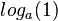
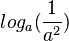
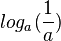
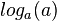
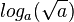
![log_a(\sqrt[5]{a})](/images/math/6/2/7/6275f4f73e05228d1ee78eff9a00be9a.png)
![log_a(\sqrt[4]{a^3})](/images/math/0/6/b/06b5e0d72b9412bfb6b60178be5a61bf.png)
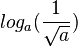
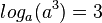
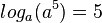
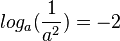
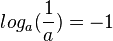
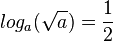
![log_a(\sqrt[5]{a})=\frac{1}{5}](/images/math/f/7/2/f7243a440fd1ad58af87bc2d1e7e2a3d.png)
![log_a(\sqrt[4]{a^3})=\frac{3}{4}](/images/math/6/e/a/6ea5cef0e0d208ab144fa286cf705c9a.png)
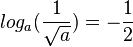
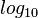 schreibt man
schreibt man 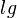
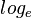 schreibt man
schreibt man 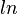 , wenn e die Eulersche Zahl e = 2, 718 281 828 459 045 235 360 287 ... ist.
, wenn e die Eulersche Zahl e = 2, 718 281 828 459 045 235 360 287 ... ist.
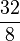 )
)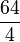 )
)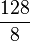 )
)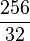 )
)

