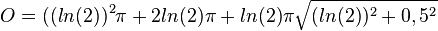M11 Aufgaben zu Logarithmus- und Exponentialfunktionen: Unterschied zwischen den Versionen
Aus RSG-Wiki
| (6 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
| + | __NOCACHE__ | ||
Buch S. 151 / 4 | Buch S. 151 / 4 | ||
| Zeile 48: | Zeile 49: | ||
b) Die Tangente in A soll parallel zu einer Geraden h mit Steigung - 0,5 sein. Also ist f'(x<sub>A</sub>) = - 0,5.<br> | b) Die Tangente in A soll parallel zu einer Geraden h mit Steigung - 0,5 sein. Also ist f'(x<sub>A</sub>) = - 0,5.<br> | ||
| − | <math>- 0,5 = -\frac{1}{2}e^{-\frac{x}{2}} \qquad | \cdot (-2)</math> | + | <math>- 0,5 = -\frac{1}{2}e^{-\frac{x}{2}} \qquad | \cdot (-2)</math><br> |
<math>1 =e^{-\frac{x}{2}} \qquad |logarithmieren</math><br> | <math>1 =e^{-\frac{x}{2}} \qquad |logarithmieren</math><br> | ||
<math>ln(1) = -\frac{x}{2}</math> ergibt <math>0 = -\frac{x}{2}</math> und <math> x = 0</math>. <br> | <math>ln(1) = -\frac{x}{2}</math> ergibt <math>0 = -\frac{x}{2}</math> und <math> x = 0</math>. <br> | ||
A(0;1) [[Datei:152-8b 1.jpg|350px]] | A(0;1) [[Datei:152-8b 1.jpg|350px]] | ||
| − | Die Tangente in B soll senkrecht zu einer Geraden k mit Steigung -2 sein. Die Tangente in B an G<sub>g</sub> hat dann die Steigung 0,5. Also ist g'(x<sub>B</sub>) = 0,5.<br< | + | Die Tangente in B soll senkrecht zu einer Geraden k mit Steigung -2 sein. Die Tangente in B an G<sub>g</sub> hat dann die Steigung 0,5. Also ist g'(x<sub>B</sub>) = 0,5.<br> |
| − | <math>0,5=\frac{1}{2}e^{x+1} \qquad |\cdot 2</math< | + | Zwei Geraden g<sub>1</sub>: y = m<sub>1</sub>x+t<sub>1</sub> und g<sub>2</sub>: y = m<sub>2</sub>x + t<sub>2</sub> stehen senkrecht zueinander, wenn m<sub>1</sub>· m<sub>2</sub> = -1 ist. |
| − | <math>1=e^{x+1} \qquad |logarithmieren</math> | + | <math>0,5=\frac{1}{2}e^{x+1} \qquad |\cdot 2</math><br> |
| + | <math>1=e^{x+1} \qquad |logarithmieren</math><br> | ||
<math> 0 = x+1</math> ergibt <math> x = -1</math> <br> | <math> 0 = x+1</math> ergibt <math> x = -1</math> <br> | ||
| − | B(-1;0,5) [[Datei:152-8b 2.jpg | + | B(-1;0,5) [[Datei:152-8b 2.jpg]] |
| + | }} | ||
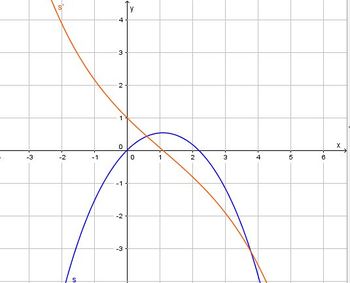
| − | Buch S. 153 / | + | Buch S. 153 / 14 |
| − | {{Lösung versteckt|1=Graph 1 gehört zu Funktion f (f ist die einzige Funktion mit D = R<sup>+</sup>.)<br> | + | {{Lösung versteckt|1=Graph 1 gehört zu Funktion f (f ist die einzige Funktion mit D = R<sup>+</sup>. Außerdem kann man den Funktionsterm vereinfachen. Es ist f(x) = 2xe<sup>ln(x)</sup>=2x<sup>2</sup> und der Graph ist eine halbe Parabel.)<br> |
| − | Graph 2 gehört zu Funktion d (d hat bei x = 0 eine Polstelle.)<br> | + | Graph 2 gehört zu Funktion d (d hat bei x = 0 eine Polstelle. )<br> |
| − | Graph 3 gehört zu Funktion a (e<sup>x</sup> wird um den Faktor 2 in y-Richtung gestreckt, ebenso in x-Richtung, also ist der Verlauf wie bei "e-Funktion" durch (0;2).)<br> | + | Graph 3 gehört zu Funktion a (e<sup>x</sup> wird um den Faktor 2 in y-Richtung gestreckt, ebenso in x-Richtung, also ist der Verlauf fast wie bei der "e-Funktion" durch (0;2).)<br> |
Graph 4 gehört zu Funktion b (-e<sup>x</sup> ist e<sup>x</sup> an der x-Achse gespiegelt und wird um 3 nach oben verschoben.)<br> | Graph 4 gehört zu Funktion b (-e<sup>x</sup> ist e<sup>x</sup> an der x-Achse gespiegelt und wird um 3 nach oben verschoben.)<br> | ||
Graph 5 gehört zu Funktion c (c ist die einzig verbleibende Funktion mit c(0) = 2.)<br> | Graph 5 gehört zu Funktion c (c ist die einzig verbleibende Funktion mit c(0) = 2.)<br> | ||
Graph 6 gehört zu Funktion e (e hat als einzige Funktion eine Nullstelle bei x = 1.) }} | Graph 6 gehört zu Funktion e (e hat als einzige Funktion eine Nullstelle bei x = 1.) }} | ||
| + | |||
| + | Buch S. 152 / 9 | ||
| + | |||
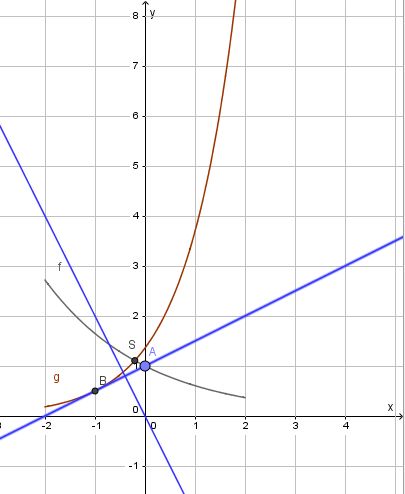
| + | {{Lösung versteckt|1=Zuerst zeichnet man den Sachverhalt.<br> | ||
| + | [[Datei:152-9.jpg]]<br> | ||
| + | Es ist f'(0) = 1, also ist die Steigung m = 1 der Tangente in (P0;1). Die Tangente hat dann die Gleichung y = x + 1. Sie schneidet die x-Achse in A(-1;0). <br> | ||
| + | Die Normale zur Tangente in P hat dann die Steigung m = -1 und sie hat die Gleichung y = -x +1. Sie schneidet die x-Achse in B(1;0).<br> | ||
| + | Das Dreieck ABP ist ein gleichschenkliges, rechtwinkliges Dreieck. Der Winkel bei P ist 90<sup>o</sup>, die Basiswinkel sind jeweils 45<sup>o</sup>. }} | ||
| + | |||
| + | Buch S. 152 / 12<br> | ||
| + | <center><ggb_applet height="400" width="600" | ||
| + | filename="152-12.ggb" /></center> | ||
| + | |||
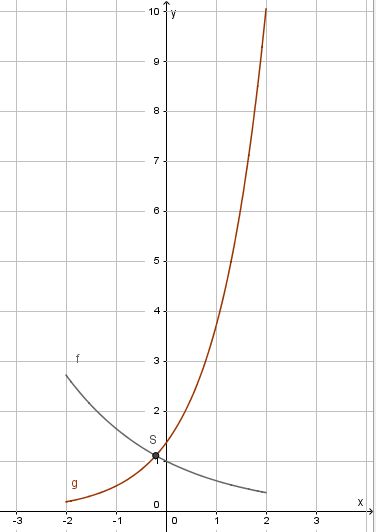
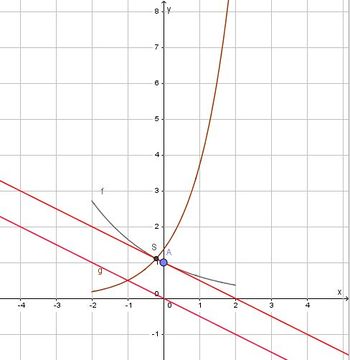
| + | {{Lösung versteckt|1=a) Die Koordinaten des Schnittpunkts B liest man gleich aus dem Diagramm ab, da beide Graphen sich bei x = 0 schneiden. Es ist B(0;1).<br> | ||
| + | Die x-Koordinate von A erhält man durch Lösen der Gleichung <math>e^{0,5x} = 4-3e^{-0,5x} </math>.<br> | ||
| + | <math>e^{0,5x} = 4-3e^{-0,5x} \qquad |\cdot e^{0,5x}</math><br> | ||
| + | <math>e^{x} = 4e^{0,5x}-3 \qquad |-4e^{0,5x}+3</math><br> | ||
| + | <math>e^{x} - 4e^{0,5x}+3 = 0 </math><br> | ||
| + | Substituiert man <math>w = e^{0,5x}</math>, dann man hat die quadratische Gleilchung <math>w^2 - 4w + 3 = 0 </math> zu lösen. Es ist <math>w^2 - 4w + 3 = (w-1)(w-3) </math> und daher hat die Gleichung <math>w^2 - 4w + 3 = 0 </math> die zwei Lösungen <math>w_1=1, w_2 = 3</math>. Die Lösung <math>w_1=1</math> führt beim Resubstituieren auf <math>0,5x = ln(1)</math>, also <math> x = 0</math>. (Lösung für B!)<br> | ||
| + | Die zweite Lösung <math>w_2 = 3</math> führt beim Resubstituieren auf <math>0,5x = ln(3)</math> und <math> x = 2\cdot ln(3) = ln(9) \approx 2,19722</math>. Also ist A(ln9; 3). | ||
| + | |||
| + | b) Es ist S(a; e<sup>0,5a</sup>) und T(a;4-3e<sup>-0,5a</sup>) und die Länge der Strecke [ST] ist <math>\overline {ST} = 4-3e^{-0,5a} - e^{0,5a}</math> (T liegt über S, also ist bei y<sub>T</sub> - y<sub>S</sub> die Differenz positiv).<br> | ||
| + | Betrachtet man nun die Funktion <math>s:a\rightarrow 4 - 3e^{-0,5a} - e^{0,5a}</math> für <math>a \in [0;ln(9)]</math>, dann muss man das Maximum von s finden. Dazu setzt man die <math>s'(a)</math> gleich 0 und erhält bei VZW +/- das gesuchte a.<br> | ||
| + | <math>s'(a)= -3e^{-0,5a}\cdot(-0,5) - e^{0,5a}\cdot 0,5 = 1,5e^{-0,5a} - 0,5e^{0,5a}</math><br> | ||
| + | <math>1,5e^{-0,5a} - 0,5e^{0,5a}= 0 \qquad |+0,5e^{0,5a}</math> | ||
| + | <math>1,5e^{-0,5a} = 0,5e^{0,5a} \qquad |\cdot 2</math><br> | ||
| + | <math>3e^{-0,5a} = e^{0,5a} \qquad |\cdot e^{0,5a}</math><br> | ||
| + | <math>3 = e^a \qquad |logarithmieren</math> <br> | ||
| + | <math>a = ln(3) \approx 1,1</math><br> | ||
| + | Lässt man sich mit GeoGebra die Graphen von s und s' zeichnen, dann hat man dieses Bild:<br> | ||
| + | [[Datei:152-12b.jpg|350px]]<br> | ||
| + | und man sieht, dass s' bei a = ln(3) eine VZW +/- hat, also hat s bei a = ln(3) ein Maximum. }} | ||
| + | |||
| + | Buch S. 154 / 18 | ||
| + | |||
| + | {{Lösung versteckt|1=<math>f: x \rightarrow 9x\cdot e^{-x}</math> mit D = R.<br> | ||
| + | a) f(0) = 9\cdot 0 \cdot e<sup>0</sup> = 0, also ist (0;0) ein Punkt von G<sub>f</sub>.<br> | ||
| + | b) Es ist <math>9x\cdot e^{-x}=9\cdot \frac{x}{e^x}</math> und nach dem Grenzwert in der [http://www.isb.bayern.de/download/13107/merkhilfe_fuer_das_fach_mathematik_standard.pdf Merkhilfe] für r = 1 ist <math>\lim_{x\to \infty}f(x)=\lim_{x\to \infty}9x\cdot e^{-x}=\lim_{x\to\infty} 9\cdot \frac{x}{e^x}=0</math> | ||
| + | |||
| + | Für den Grenzwert <math>x \to -\infty</math> ist <math>\lim_{x\to -\infty}f(x) =\ </math>" <math>9\cdot(-\infty) \cdot \infty</math> "<math>= -\infty</math> | ||
| + | |||
| + | c) Es ist <math>f'(x)=9e^{-x}+9x\cdot e^{-x}\cdot(-1)=9e^{-x}(1-x)</math><br> | ||
| + | Da stets <math>e^{-x} > 0</math> ist, ist <math>f'(x)=0</math> für 1 - x = 0 und x = 1. <br> | ||
| + | Da y = 1 - x eine fallende Gerade mit Nullstelle x = 1 ist, ist dort der VZW +/- und damit ist bei x = 0 ein Maximum, G<sub>f</sub> hat in (1;<math>\frac{9}{e}</math>) einen Hochpunkt. | ||
| + | |||
| + | d)<br> | ||
| + | [[Datei:154-18.jpg|300px]] | ||
| + | |||
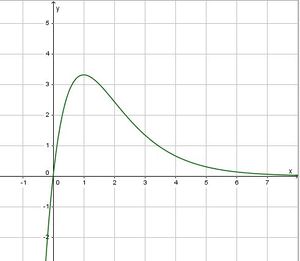
| + | e) Das Bild zu dieser Aufgabe schaut so aus:<br> | ||
| + | [[Datei:154-18e.jpg|300px]]<br> | ||
| + | Aufstellen der Gleichung der Tangente:<br> | ||
| + | <math>f(2) = 18\cdot e^{-2} = \frac{18}{e^2}\approx 2,436</math>. <br> | ||
| + | <math>f'(2)=9e^{-2}(1-2)=\frac{-9}{e^2}\approx 1,218</math>. <br> | ||
| + | Ansatz: <math> y = mx + t</math> mit <math>m = \frac{-9}{e^2}</math> und <math>P(2;\frac{18}{e^2}</math> erhält man<br> | ||
| + | <math> \frac{18}{e^2} = \frac{-9}{e^2}\cdot 2 + t </math><br> | ||
| + | <math> t = \frac{36}{e^2} \approx 4,872</math><br> | ||
| + | Die Gleichung der Tangente t ist <math> y=\frac{-9}{e^2}x + \frac{36}{e^2}</math>. | ||
| + | |||
| + | Der Schnittpunkt mit der y-Achse ist <math>(0;\frac{36}{e^2}</math>), der Schnittpunkt mit der x-Achse ist (4;0) | ||
| + | |||
| + | Der Flächeninhalt <math>a_1</math> des Dreiecks TIP ist <math>A_1=\frac{1}{2}\cdot 2 \cdot (\frac{36}{e^2} - \frac{18}{e^2})\approx 2,436</math><br> | ||
| + | Der Flächeninhalt <math>A</math> des Dreiecks OST ist <math>A=\frac{1}{2}\cdot 4\cdot \frac{36}{e^2}\approx 9,744</math><br> | ||
| + | Das Flächenverhältnis ist <math>\frac{A_1}{A}=\frac{1}{4}</math>, also nimmt die Fläche des Dreicks TIP 25% der Fläche des Dreiecks OST ein.<br> | ||
| + | Diese Aufgabe hätte man auch leichter mit dem Strahlensatz lösen können. Die "kleine waagrechte Strecke" [IP] hat die Länge 2, die "große waagrechte Strecke" [OS] hat die Länge 4. Daher ist der Faktor 2 und für das Verhältnis "Fläche kleines Dreieck" : "Fläche großes Dreieck" = 1 : 2<sup>2</sup> = 1 : 4. Damit hat man das gleiche Ergebnis. | ||
| + | |||
| + | f) Die Situation schaut so aus:<br> | ||
| + | [[Datei:154-18f.jpg|300px]]<br> | ||
| + | Der Flächeninhalt des Dreiecks OLA ist <math>A(s) = \frac{1}{2}\cdot s \cdot f(s) = \frac{1}{2}\cdot s \cdot 9se^{-s}=\frac{9}{2}\cdot s^2\cdot e^{-s}</math><br> | ||
| + | <math>A'(s) = \frac{9}{2}[2se^{-s}+s^2e^{-s}(-1)]=\frac{9}{2}e^{-s}s(2-s)</math> <br> | ||
| + | Es ist <math>\frac{9}{2}e^{-s} > 0</math> und <math>s (2-s)=2s-s^2</math> ist der Term einer nach unten geöffneten Parabel mit den Nullstellen 0 und 2. Da die Parabel nach unten geöffnet ist, ist bei s = 0 ein VZW -/+ und bei s = 2 ein VZW +/-. Daher hat A bei x = 2 ein Maximum und es ist <math>A(2) = 18e^{-2}=\frac{18}{e^2}\approx 2,436</math>. }} | ||
| + | |||
| + | Buch S. 159 / 6 | ||
| + | |||
| + | {{Lösung versteckt|1=<math>f(x) = (1-ln(x))^2</math> D = R<sup>+</sup><br> | ||
| + | Gemeinsame Punkte mit der x-Achse: <math>(1-ln(x))^2=0</math> ist erfüllt für <math>1=ln(x)</math>, also <math>x=e</math>.<br> | ||
| + | <math>f'(x)=2(1-ln(x))\cdot (-\frac{1}{x})=\frac{2(ln(x)-1)}{x}</math><br> | ||
| + | Für x<math>\in</math>R<sup>+</sup> ist der Nenner positiv. Für das Vorzeichen von f' muss man also den Zähler betrachten. <br> | ||
| + | Für x < e ist ln(x) < 1 und ln(x) - 1 < 0, also ist f'(x) < 0 für x < e und f ist in ]0;e] streng monoton abnehmend. <br> | ||
| + | Für x > e ist ln(x) > 1 und ln(x) - 1 > 0, also ist f'(x) > 0 für x > e und f ist in ]e;<math>\infty</math>[ streng monoton zunehmend. <br> | ||
| + | Da x = e die einzige Nullstelle von f' ist und einen VZW -/+ hat ist bei (e;0) ein Minimum und G<sub>f</sub> hat in (e;0) einen Tiefpunkt. <br> | ||
| + | Die positive y-Achse ist Asymptote für <math>x \to 0</math>. }} | ||
| + | |||
| + | Buch S. 160 / 10 | ||
| + | |||
| + | {{Lösung versteckt|1=<math>f(x) = ln(x^2+\frac{1}{4}) - ln(x)</math>, D = R<sup>+</sup><br> | ||
| + | a) Es ist <math>ln(x^2+\frac{1}{4}) - ln(x)=ln \left ( \frac{x^2 + 0,25}{x} \right )</math><br> | ||
| + | Gemeinsame Punkte mit der x-Achse erhält man, wenn <math>f(x) = 0 </math> ist. Es ist <math>ln \left (\frac{x^2+0,25}{x} \right )=0</math> für <math>\frac{x^2+0,25}{x} = 1</math>.<br> | ||
| + | <math>x^2+0,25 = x</math> ist eine quadratische Gleichung.<br> | ||
| + | <math>x^2 - x + 0,25 = 0</math> ergibt mit der binomischen Formel <math>(x-0,5)^2 = 0</math>, also ist x = 0,5 (doppelte) Nullstelle von f. | ||
| + | |||
| + | <math>f'(x) = \frac{1}{x^2+0,25} \cdot 2x - \frac{1}{x}=\frac{2x}{x^2+0,25}-\frac{1}{x}=\frac{2x^2 -(x^2+0,25)}{x(x^2+0,25)}=\frac{x^2-0,25}{x(x^2+0,25)}</math><br> | ||
| + | <math>f'(x) = 0</math> ist <math>\frac{x^2-0,25}{x(x^2+0,25)}=0</math>. Da der Nenner für positive x stets positiv ist, muss man nur den Zähler betrachten. Es ist <math>x^2-0,25=(x+0,5)(x-0,5)</math>. Der Zähler ist 0 für x = - 0,5 und x = 0,5. -0,5 ist nicht in D, also ist <math>f'(x)=0</math> für x = 0,5. <br> | ||
| + | Es ist <math>f(0,5)=ln(\frac{0,5^2+0,25}{0,5})=ln(1)=0</math><br> | ||
| + | Der Zähler ist eine nach oben geöffnete Parabel, die bei der rechten Nullstelle einen VZW -/+ hat. (Der Nenner ist positiv!). Daher hat f' bei x = 0,5 einen VZW -/+ und f hat bei x = 0,5 ein Minimum, G<sub>f</sub> bei (0,5;0) einen Tiefpunkt. | ||
| + | |||
| + | b) <math>\lim_{x\to 0+}[ln(x^2+\frac{1}{4}) - ln(x)]=\infty</math>, da ln(0,25) < 0 und <math>lim_{x\to 0}ln(x)=-\infty</math> ist.<br> | ||
| + | Die positive y-Achse ist für <math>x \to 0+</math> Asymptote. | ||
| + | |||
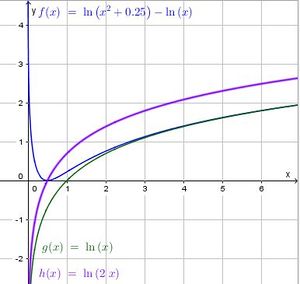
| + | <math>\lim_{x\to \infty}[ln(x^2+\frac{1}{4}) - ln(x)] =\lim_{x\to \infty}ln(\frac{x^2+0,25}{x}=\infty</math>, da der Grad des Zählerpolynoms 2 ist und damit größer als der Grad des Nennerpolynoms. Der Bruch geht für <math>x\to \infty</math> auch gegen <math>\infty</math>.<br> | ||
| + | Der Graph hat für <math>x\to \infty</math> keine Asymptote. | ||
| + | |||
| + | <math>\lim_{x\to \infty}[ln(x^2+\frac{1}{4}) - ln(x) -ln(x)]=\lim_{x\to \infty}[ln(x^2+0,25)-2ln(x)=\lim_{x\to \infty}\frac{x^2+0,25}{x^2}=ln(1)=0</math>, da der Grad des Zählerpolynoms gleich dem Grad des Nennerpolynoms ist. Damit geht der Bruch für <math>x\to \infty</math> gegen 1 .<br> | ||
| + | Die positive x-Achse ist für <math>x\to \infty</math> Asymptote. | ||
| + | |||
| + | c) <br> | ||
| + | [[Datei:160-10c.jpg|300px]] | ||
| + | |||
| + | d) g(x) = ln(x). Wenn im Argument 2x steht, dann wird der Graph von g um den Faktor 2 in x-Richtung gestaucht. <br> | ||
| + | [[Datei:160-10d.jpg|300px]]<br> | ||
| + | Den Schnittpunkt von G<sub>h</sub> und G<sub>f</sub> erhält man, wenn man <math>ln(2x) = ln(\frac{x^2+0,25}{x})</math> löst. Wenn die beiden Logarithmen gleich sind, müssen auch ihre Argumente gleich sein und man muss die Gleichung <math>2x = \frac{x^2+0,25}{x}</math> lösen.<br> | ||
| + | <math>2x = \frac{x^2+0,25}{x} \qquad |\cdot x</math> <br> | ||
| + | <math>2x^2 = x^2 + 0,25 \qquad |-x^2</math><br> | ||
| + | <math>x^2 = 0,25</math><br> | ||
| + | <math>x_{1,2}=\pm 0,5</math><br> | ||
| + | Da D = R<sup>+</sup> ist, hat man nur die Lösung x = 0,5 und der Schnittpunkt ist (0,5;0) (x = 0,5 ist Nullstelle, was man in a) gezeigt hat!)<br> | ||
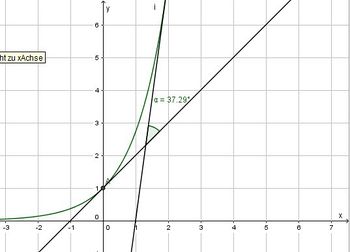
| + | Den Schnittwinkel <math>\varphi</math> erhält man, wenn man <math>tan(\varphi_1)=h'(0,5)</math> und <math>tan(\varphi_2)=f'(0,5)</math> bestimmt und dann <math>\varphi = \varphi_1 - \varphi_2</math> berechnet.<br> | ||
| + | Es ist <math>h'(x)=\frac{1}{x}</math> , <math>tan(\varphi_1)=h'(0,5)=2</math> und <math>\varphi_1=63,4^o</math>.<br> | ||
| + | <math>tan(\varphi_2)=f'(0,5)=0</math> und <math>\varphi_2=0^o</math>. <br> | ||
| + | Also erhält man <math>\varphi = 63,4^o</math>. | ||
| + | |||
| + | e)<br> [[Datei:160-10e.jpg]]<br> | ||
| + | Wenn das Trapez um die x-Ache rotiert entsteht ein Zylinder, bei dem rechts ein Kegel herausgeschnitten ist. Die Grundfläche des Zylinders ist ein Kreis mit Mittelpunkt (0;0) und Radius r = ln(2). Die Höhe des Zylinders ist h = 1, also hat der Zylinder das Volumen <math>V_Z = r^2\pi \cdot h = (ln(2))^2 \pi</math>.<br> | ||
| + | Der Kegel, der recht herausgenommen wird hat ebenfalls den Radius r = ln(2), aber die Höhe h<sub>K</sub> = 0,5. Sein Volumen ist <math>V_K=\frac{1}{3}(ln(2))^2\cdot 0,5 \cdot \pi=\frac{(ln(2))^2}{6}\cdot \pi</math>.<br> | ||
| + | Damit hat der Rotationskörper das Volumen <math>V = V_Z - V_K = (ln(2))^2 \cdot \pi- \frac{(ln(2))^2}{6}\cdot \pi=\frac{5(ln(2))^2}{6}\cdot \pi</math>. | ||
| + | |||
| + | Die Oberfläche des Rotationskörpers setzt sich aus der Grundfläche des Zylinders, seiner Mantelfläche und der Mantelfläche des Kegel zusammen. Es ist <math>G = ((ln(2))^2\pi</math>, <math>M_Z=2r_Z\pi h_Z=2ln(2)\pi</math> und <math>M_K=r_K \pi m=ln(2)\pi\sqrt{(ln(2))^2 + 0,5^2}</math>.<br> | ||
| + | Damit ist <math>O = ((ln(2))^2\pi + 2ln(2)\pi + ln(2)\pi\sqrt{(ln(2))^2 + 0,5^2}</math> | ||
| + | |||
| + | |||
| + | |||
| + | }} | ||
Aktuelle Version vom 20. April 2021, 16:29 Uhr
Buch S. 151 / 4
Buch S. 152 / 7a
Buch S. 152 / 8
Buch S. 153 / 14
Buch S. 152 / 9
Buch S. 152 / 12
Buch S. 154 / 18
Buch S. 159 / 6
Buch S. 160 / 10
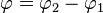 bildet, wenn
bildet, wenn 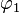 der Schnittwinkel von t1 mit der Waagrechten im Schnittpunkt und
der Schnittwinkel von t1 mit der Waagrechten im Schnittpunkt und 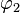 der Schnittwinkel von t2 mit der Waagrechten im Schnittpunkt ist.
der Schnittwinkel von t2 mit der Waagrechten im Schnittpunkt ist.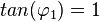 , also ist
, also ist 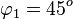 .
. 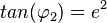 , also ist
, also ist 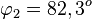
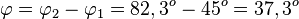 .
.
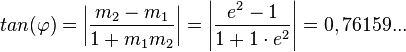 ergibt
ergibt 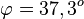 .
.
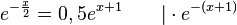
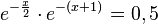

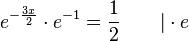

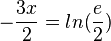
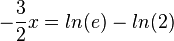
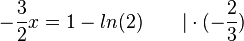
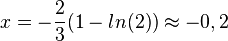
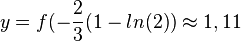 , also S(-0,2; 1,11) (näherungsweise, aber genügend genau!)
, also S(-0,2; 1,11) (näherungsweise, aber genügend genau!)
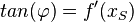 .
.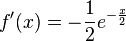 und
und 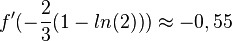 .
.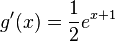 und
und 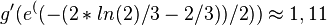 .
.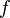 ist
ist 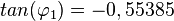 und
und 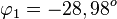 und
und ist
ist  und
und  .
.  .
.
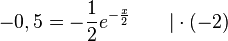
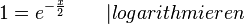
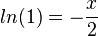 ergibt
ergibt 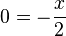 und
und 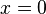 .
. 
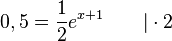

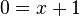 ergibt
ergibt 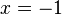
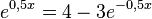 .
.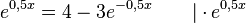

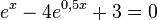
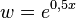 , dann man hat die quadratische Gleilchung
, dann man hat die quadratische Gleilchung 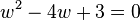 zu lösen. Es ist
zu lösen. Es ist 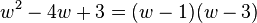 und daher hat die Gleichung
und daher hat die Gleichung 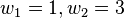 . Die Lösung
. Die Lösung 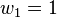 führt beim Resubstituieren auf
führt beim Resubstituieren auf  , also
, also 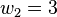 führt beim Resubstituieren auf
führt beim Resubstituieren auf 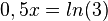 und
und 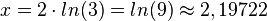 . Also ist A(ln9; 3).
. Also ist A(ln9; 3).
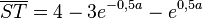 (T liegt über S, also ist bei yT - yS die Differenz positiv).
(T liegt über S, also ist bei yT - yS die Differenz positiv).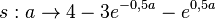 für
für ![a \in [0;ln(9)]](/images/math/6/8/3/683526e7906cfa2c08a68415cb17f301.png) , dann muss man das Maximum von s finden. Dazu setzt man die
, dann muss man das Maximum von s finden. Dazu setzt man die 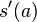 gleich 0 und erhält bei VZW +/- das gesuchte a.
gleich 0 und erhält bei VZW +/- das gesuchte a.

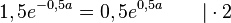
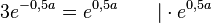
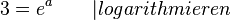
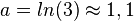
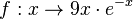 mit D = R.
mit D = R.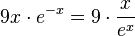 und nach dem Grenzwert in der
und nach dem Grenzwert in der 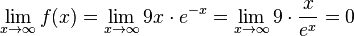
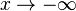 ist
ist 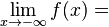 "
" 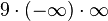 "
"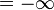
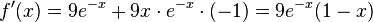
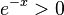 ist, ist
ist, ist 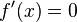 für 1 - x = 0 und x = 1.
für 1 - x = 0 und x = 1.  ) einen Hochpunkt.
) einen Hochpunkt.

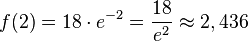 .
. 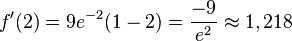 .
. 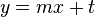 mit
mit 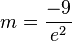 und
und 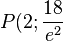 erhält man
erhält man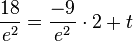
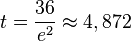
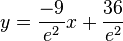 .
.
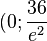 ), der Schnittpunkt mit der x-Achse ist (4;0)
), der Schnittpunkt mit der x-Achse ist (4;0)
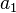 des Dreiecks TIP ist
des Dreiecks TIP ist 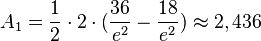
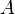 des Dreiecks OST ist
des Dreiecks OST ist 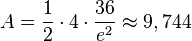
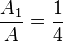 , also nimmt die Fläche des Dreicks TIP 25% der Fläche des Dreiecks OST ein.
, also nimmt die Fläche des Dreicks TIP 25% der Fläche des Dreiecks OST ein.
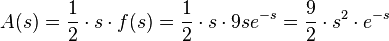
![A'(s) = \frac{9}{2}[2se^{-s}+s^2e^{-s}(-1)]=\frac{9}{2}e^{-s}s(2-s)](/images/math/a/e/8/ae86fa5ca668086e103dc7c83ff73d63.png)
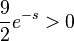 und
und  ist der Term einer nach unten geöffneten Parabel mit den Nullstellen 0 und 2. Da die Parabel nach unten geöffnet ist, ist bei s = 0 ein VZW -/+ und bei s = 2 ein VZW +/-. Daher hat A bei x = 2 ein Maximum und es ist
ist der Term einer nach unten geöffneten Parabel mit den Nullstellen 0 und 2. Da die Parabel nach unten geöffnet ist, ist bei s = 0 ein VZW -/+ und bei s = 2 ein VZW +/-. Daher hat A bei x = 2 ein Maximum und es ist 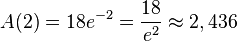 .
.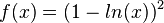 D = R+
D = R+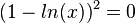 ist erfüllt für
ist erfüllt für 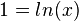 , also
, also 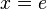 .
.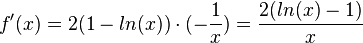
 R+ ist der Nenner positiv. Für das Vorzeichen von f' muss man also den Zähler betrachten.
R+ ist der Nenner positiv. Für das Vorzeichen von f' muss man also den Zähler betrachten. 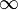 [ streng monoton zunehmend.
[ streng monoton zunehmend. 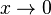 .
.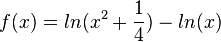 , D = R+
, D = R+
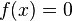 ist. Es ist
ist. Es ist 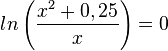 für
für 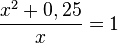 .
. ist eine quadratische Gleichung.
ist eine quadratische Gleichung.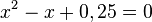 ergibt mit der binomischen Formel
ergibt mit der binomischen Formel 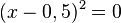 , also ist x = 0,5 (doppelte) Nullstelle von f.
, also ist x = 0,5 (doppelte) Nullstelle von f.
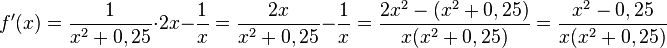
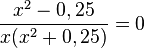 . Da der Nenner für positive x stets positiv ist, muss man nur den Zähler betrachten. Es ist
. Da der Nenner für positive x stets positiv ist, muss man nur den Zähler betrachten. Es ist  . Der Zähler ist 0 für x = - 0,5 und x = 0,5. -0,5 ist nicht in D, also ist
. Der Zähler ist 0 für x = - 0,5 und x = 0,5. -0,5 ist nicht in D, also ist 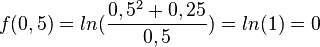
![\lim_{x\to 0+}[ln(x^2+\frac{1}{4}) - ln(x)]=\infty](/images/math/8/f/3/8f35c93b9bb3d1bd559a0409d48ab8b5.png) , da ln(0,25) < 0 und
, da ln(0,25) < 0 und  ist.
ist.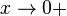 Asymptote.
Asymptote.
![\lim_{x\to \infty}[ln(x^2+\frac{1}{4}) - ln(x)] =\lim_{x\to \infty}ln(\frac{x^2+0,25}{x}=\infty](/images/math/9/7/8/978116f59c7f1c3700f0089f84b2e8f6.png) , da der Grad des Zählerpolynoms 2 ist und damit größer als der Grad des Nennerpolynoms. Der Bruch geht für
, da der Grad des Zählerpolynoms 2 ist und damit größer als der Grad des Nennerpolynoms. Der Bruch geht für 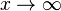 auch gegen
auch gegen ![\lim_{x\to \infty}[ln(x^2+\frac{1}{4}) - ln(x) -ln(x)]=\lim_{x\to \infty}[ln(x^2+0,25)-2ln(x)=\lim_{x\to \infty}\frac{x^2+0,25}{x^2}=ln(1)=0](/images/math/e/9/9/e99faacae52c6f9b9ac9705815ff1f07.png) , da der Grad des Zählerpolynoms gleich dem Grad des Nennerpolynoms ist. Damit geht der Bruch für
, da der Grad des Zählerpolynoms gleich dem Grad des Nennerpolynoms ist. Damit geht der Bruch für 
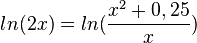 löst. Wenn die beiden Logarithmen gleich sind, müssen auch ihre Argumente gleich sein und man muss die Gleichung
löst. Wenn die beiden Logarithmen gleich sind, müssen auch ihre Argumente gleich sein und man muss die Gleichung 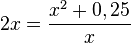 lösen.
lösen.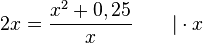
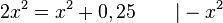
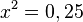
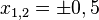
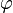 erhält man, wenn man
erhält man, wenn man  und
und 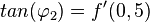 bestimmt und dann
bestimmt und dann 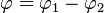 berechnet.
berechnet.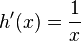 ,
, 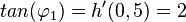 und
und 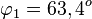 .
.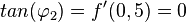 und
und  .
. 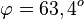 .
.

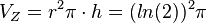 .
.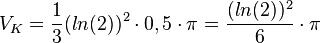 .
. .
.
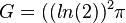 ,
, 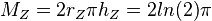 und
und 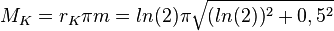 .
.