M11 Aufgaben zu Logarithmus- und Exponentialfunktionen: Unterschied zwischen den Versionen
Aus RSG-Wiki
| Zeile 101: | Zeile 101: | ||
[[Datei:152-12b.jpg|350px]]<br> | [[Datei:152-12b.jpg|350px]]<br> | ||
und man sieht, dass s' bei a = ln(3) eine VZW +/- hat, also hat s bei a = ln(3) ein Maximum. }} | und man sieht, dass s' bei a = ln(3) eine VZW +/- hat, also hat s bei a = ln(3) ein Maximum. }} | ||
| + | |||
| + | Buch S. 154 / 18 | ||
| + | |||
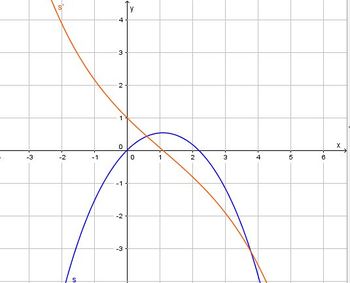
| + | {{Lösung versteckt|1=<math>f: x \rightarrow 9x\cdot e^{-x}</math> mit D = R.<br> | ||
| + | a) f(0) = 9\cdot 0 \cdot e<sup>0</sup> = 0, also ist (0;0) ein Punkt von G<sub>f</sub>.<br> | ||
| + | b) Es ist <math>9x\cdot e^{-x}=9\cdot \frac{x}{e^x}</math> und nach dem Grenzwert in der [http://www.isb.bayern.de/download/13107/merkhilfe_fuer_das_fach_mathematik_standard.pdf Merkhilfe] für r = 1 ist <math>\lim_{x\to \infty}f(x)=\lim_{x\to \infty}9x\cdot e^{-x}=\lim_{x\to\infty} 9\cdot \frac{x}{e^x}=0</math> | ||
| + | |||
| + | Für den Grenzwert <math>x \to -\infty</math> ist <math>\lim_{x\to -\infty}f(x) =\ </math>" <math>9\cdot(-\infty) \cdot \infty</math> "<math>= -\infty</math> | ||
| + | |||
| + | c) Es ist <math>f'(x)=9e^{-x}+9x\cdot e^{-x}\cdot(-1)=9e^{-x}(1-x)</math><br> | ||
| + | Da stets <math>e^{-x} > 0</math> ist, ist <math>f'(x)=0</math> für 1 - x = 0 und x = 1. <br> | ||
| + | Da y = 1 - x eine fallende Gerade mit Nullstelle x = 1 ist, ist dort der VZW +/- und damit ist bei x = 0 ein Maximum, G<sub>f</sub> hat in (1;<math>\frac{9}{e}</math>) einen Hochpunkt. | ||
| + | |||
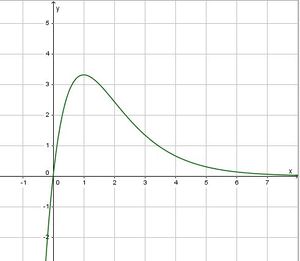
| + | d)<br> | ||
| + | [[Datei:154-18.jpg|300px]] | ||
| + | |||
| + | e) Das Bild zu dieser Aufgabe schaut so aus:<br> | ||
| + | [[Datei:154-18e.jpg|300px]]<br> | ||
| + | Aufstellen der Gleichung der Tangente:<br> | ||
| + | <math>f(2) = 18\cdot e^{-2} = \frac{18}{e^2}\approx 2,436</math>. <br> | ||
| + | <math>f'(2)=9e^{-2}(1-2)=\frac{-9}{e^2}\approx 1,218</math>. <br> | ||
| + | Ansatz: <math> y = mx + t</math> mit <math>m = \frac{-9}{e^2}</math> und <math>P(2;\frac{18}{e^2}</math> erhält man<br> | ||
| + | <math> \frac{18}{e^2} = \frac{-9}{e^2}\cdot 2 + t </math><br> | ||
| + | <math> t = \frac{36}{e^2} \approx 4,872</math><br> | ||
| + | Die Gleichung der Tangente t ist <math> y=\frac{-9}{e^2}x + \frac{36}{e^2}</math>. | ||
| + | |||
| + | Der Schnittpunkt mit der y-Achse ist <math>(0;\frac{36}{e^2}</math>), der Schnittpunkt mit der x-Achse ist (4;0) | ||
| + | |||
| + | Der Flächeninhalt <math>a_1</math> des Dreiecks TIP ist <math>A_1=\frac{1}{2}\cdot 2 \cdot (\frac{36}{e^2} - \frac{18}{e^2})\approx 2,436</math><br> | ||
| + | Der Flächeninhalt <math>A</math> des Dreiecks OST ist <math>A=\frac{1}{2}\cdot 4\cdot \frac{36}{e^2}\approx 9,744</math><br> | ||
| + | Das Flächenverhältnis ist <math>\frac{A_1}{A}=\frac{1}{4}</math>, also nimmt die Fläche des Dreicks TIP 25% der Fläche des Dreiecks OST ein.<br> | ||
| + | Diese Aufgabe hätte man auch leichter mit dem Strahlensatz lösen können. Die "kleine waagrechte Strecke" [IP] hat die Länge 2, die "große waagrechte Strecke" [OS] hat die Länge 4. Daher ist der Faktor 2 und für das Verhältnis "Fläche kleines Dreieck" : "Fläche großes Dreieck" = 1 : 2<sup>2</sup> = 1 : 4. Damit hat man das gleiche Ergebnis. | ||
| + | |||
| + | f) Die Situation schaut so aus:<br> | ||
| + | [[Datei:154-18f.jpg|300px]]<br> | ||
| + | Der Flächeninhalt des Dreiecks OLA ist <math>A(s) = \frac{1}{2}\cdot s \cdot f(s) = \frac{1}{2}\cdot s \cdot 9se^{-s}=\frac{9}{2}\cdot s^2\cdot e^{-s}</math><br> | ||
| + | <math>A'(s) = \frac{9}{2}[2se^{-s}+s^2e^{-s}(-1)]=\frac{9}{2}e^{-s}s(2-s)</math> }} | ||
Version vom 16. April 2021, 15:21 Uhr
Buch S. 151 / 4
Buch S. 152 / 7a
Buch S. 152 / 8
Buch S. 153 / 14
Buch S. 152 / 9
Buch S. 152 / 12
Buch S. 154 / 18
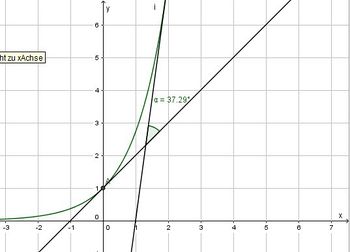
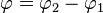 bildet, wenn
bildet, wenn 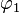 der Schnittwinkel von t1 mit der Waagrechten im Schnittpunkt und
der Schnittwinkel von t1 mit der Waagrechten im Schnittpunkt und 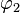 der Schnittwinkel von t2 mit der Waagrechten im Schnittpunkt ist.
der Schnittwinkel von t2 mit der Waagrechten im Schnittpunkt ist.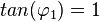 , also ist
, also ist 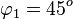 .
. 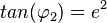 , also ist
, also ist 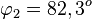
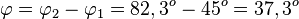 .
.
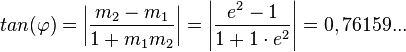 ergibt
ergibt 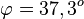 .
.
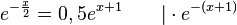
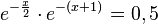

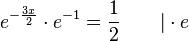

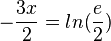
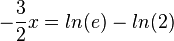
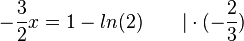
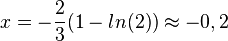
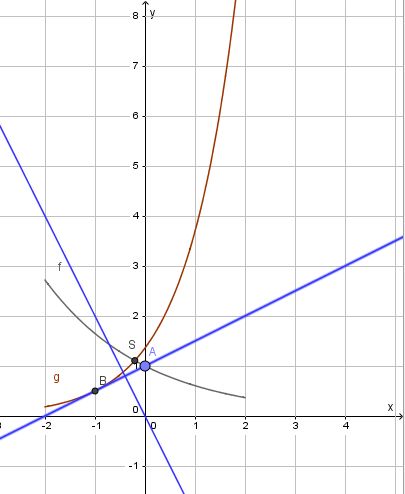
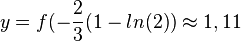 , also S(-0,2; 1,11) (näherungsweise, aber genügend genau!)
, also S(-0,2; 1,11) (näherungsweise, aber genügend genau!)
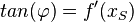 .
.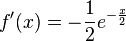 und
und 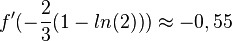 .
.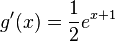 und
und 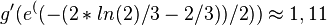 .
.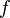 ist
ist 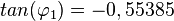 und
und 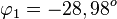 und
und ist
ist  und
und 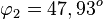 .
. 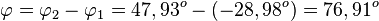 .
.
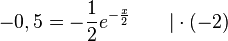
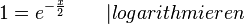
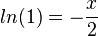 ergibt
ergibt 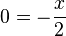 und
und 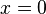 .
. 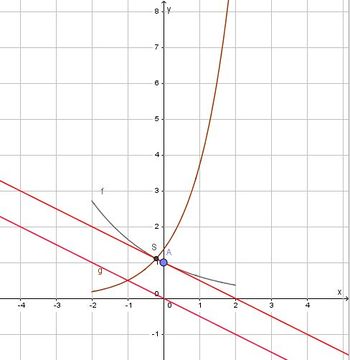
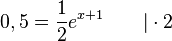

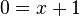 ergibt
ergibt 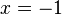
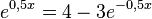 .
.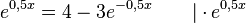

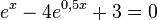
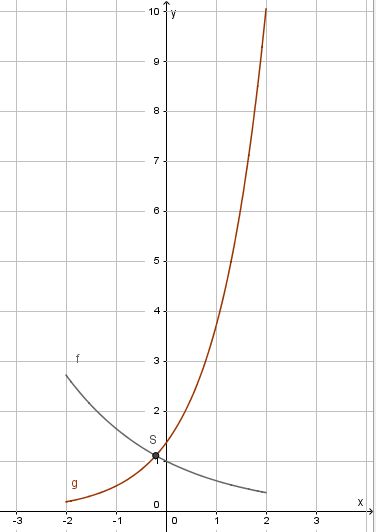
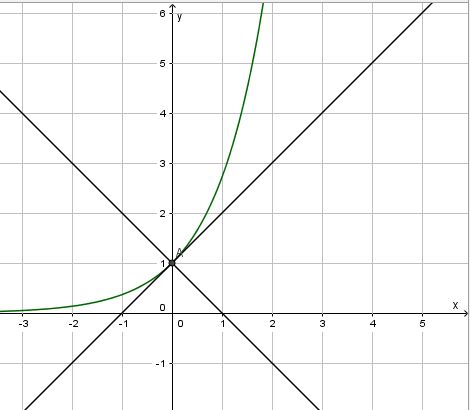
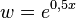 , dann man hat die quadratische Gleilchung
, dann man hat die quadratische Gleilchung 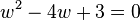 zu lösen. Es ist
zu lösen. Es ist 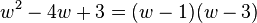 und daher hat die Gleichung
und daher hat die Gleichung 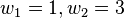 . Die Lösung
. Die Lösung 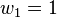 führt beim Resubstituieren auf
führt beim Resubstituieren auf  , also
, also 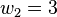 führt beim Resubstituieren auf
führt beim Resubstituieren auf 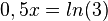 und
und 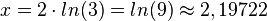 . Also ist A(ln9; 3).
. Also ist A(ln9; 3).
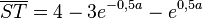 (T liegt über S, also ist bei yT - yS die Differenz positiv).
(T liegt über S, also ist bei yT - yS die Differenz positiv).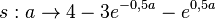 für
für ![a \in [0;ln(9)]](/images/math/6/8/3/683526e7906cfa2c08a68415cb17f301.png) , dann muss man das Maximum von s finden. Dazu setzt man die
, dann muss man das Maximum von s finden. Dazu setzt man die 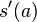 gleich 0 und erhält bei VZW +/- das gesuchte a.
gleich 0 und erhält bei VZW +/- das gesuchte a.

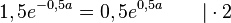
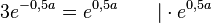
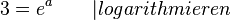
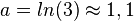
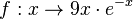 mit D = R.
mit D = R.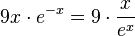 und nach dem Grenzwert in der
und nach dem Grenzwert in der 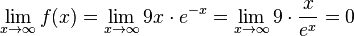
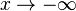 ist
ist 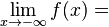 "
" 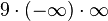 "
"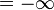
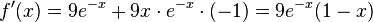
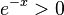 ist, ist
ist, ist 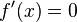 für 1 - x = 0 und x = 1.
für 1 - x = 0 und x = 1.  ) einen Hochpunkt.
) einen Hochpunkt.

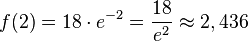 .
. 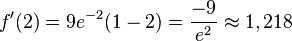 .
. 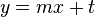 mit
mit 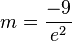 und
und 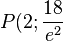 erhält man
erhält man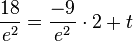
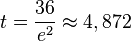
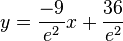 .
.
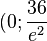 ), der Schnittpunkt mit der x-Achse ist (4;0)
), der Schnittpunkt mit der x-Achse ist (4;0)
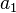 des Dreiecks TIP ist
des Dreiecks TIP ist 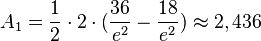
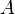 des Dreiecks OST ist
des Dreiecks OST ist 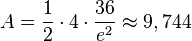
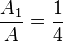 , also nimmt die Fläche des Dreicks TIP 25% der Fläche des Dreiecks OST ein.
, also nimmt die Fläche des Dreicks TIP 25% der Fläche des Dreiecks OST ein.
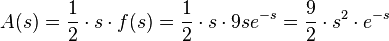
![A'(s) = \frac{9}{2}[2se^{-s}+s^2e^{-s}(-1)]=\frac{9}{2}e^{-s}s(2-s)](/images/math/a/e/8/ae86fa5ca668086e103dc7c83ff73d63.png)

