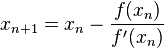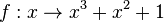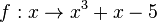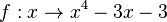M11 Das Newtonsche Iterationsverfahren: Unterschied zwischen den Versionen
| Zeile 43: | Zeile 43: | ||
{{Aufgaben-blau|4|2=Bearbeiten Sie im Buch S. 84/10 und 84/11}} | {{Aufgaben-blau|4|2=Bearbeiten Sie im Buch S. 84/10 und 84/11}} | ||
| + | |||
| + | {{Lösung versteckt|1=84/11 <math>f: x \rightarrow \frac{1}{4}x^4-\frac{4}{3}x^3+2x^2</math><br> | ||
| + | G<sub>f</sub> hat Schnittpunkt mit der y-Achse (0;0), da f(0)=0 ist und <br> | ||
| + | Schnittpunkt mit der x-Achse (0;0), da <math>\frac{1}{4}x^4-\frac{4}{3}x^3+2x^2=0</math><br> | ||
| + | <math>x^2(\frac{1}{4}x^2-\frac{4}{3}x+2)=0</math><br><br> | ||
| + | Ein Produkt ist 0, wenn ein Faktor 0 ist, also <math>x=0</math> oder <math>\frac{1}{4}x^2-\frac{4}{3}x+2=0</math>. | ||
| + | Die quadratische Gleichung hat keine Lösung, da ihre Diskriminante <math>D=\left ( \frac{4}{3} \right )^2-4\cdot \frac{1}{4} \cdot 2=\frac{16}{9}-2 <0</math><br> | ||
| + | Also ist x=0 doppelte Nullstelle.<br> | ||
| + | Für die Monotonietabelle braucht man <math>f'</math>. Es ist <math>f'(x)=x^3-4x^2+4x=x(x-2)^2</math><br> | ||
| + | <math>f'(x)=0</math> für <math> x_1=0 , x_2=2</math>, wobei x<sub>2</sub>=2 zweifach ist.<br> | ||
| + | G<sub>f'</sub> ist der Graph eines Polynoms 3. Grades mit Nullstelle bei x<sub>1</sub>=0 und x<sub>2</sub>=2 (doppelt), also hat man bei x<sub>2</sub>=2 keinen Vorzeichenwechsel. <br> | ||
| + | Monotonietabelle:<br> | ||
| + | Für x < 0 ist f'(x) < 0 und G<sub>f</sub> ist streng monoton fallend.<br> | ||
| + | Für x = 0 ist f'(0) = 0 und (0;0) ist wegen VZW -/+ ein TP.<br> | ||
| + | Für 0 < x < 2 ist f'(x) > 0 und G<sub>f</sub> ist streng monoton steigend.<br> | ||
| + | Für x = 2 ist f'(2) = 0 und G<sub>f</sub> hat dort einen Terrassenpunkt, da kein VZW.<br> | ||
| + | Für x > 0 ist f'(x) > 0 und G<sub>f</sub> ist streng monoton steigend. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | }} | ||
Version vom 15. Dezember 2020, 11:15 Uhr
Für lineare und quadratische Funktionen hat man zur Bestimmung der Nullstellen Gleichungen zu lösen. Bei quadratischen Funktionen gibt es hierzu die Lösungsformel. Für Polynome höheren Grades kann man meist nur Nullstellen erraten und dann per Polynomdivision versuchen auf ein Polynom 2. Grades zu kommen.
Bei vielen Funktionen hat man Probleme die Nullstellen zu bestimmen. Oftmals reicht es aus, wenn man einen Näherungswert hat. Ein Verfahren um einen Näherungswert für die Nullstelle einer Funktion zu finden ist das Newtonsche Iterationsverfahren.
|
Newtonsche Iterationsformel Diese Formel steht auch in der Merkhilfe |
a) x1 = 3,2235
Diese Lösung soll nochmals ausführlich dargestellt werden. Man erhält x1 indem man im Punkt P(x0),f(x0) auf dem Graphen von f die Tangente macht.
Es ist f(5) = 53+52+1=151.
Die Steigung der Tangente erhält man durch f'(5).
Die Ableitungsfunktion f' ist durch f(x) = 3x2+2x gegeben. Es ist f'(5) = 85.
Den Schnittpunkt der Tangente mit der x-Achse ist nun x1 und x1 erhält man nun durch die Formel 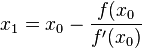 .
.
Es ist also 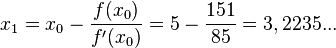
b) x1 = 3,3552
a) x26 = - 1,46557
b) x6 = 1,51598
84/11 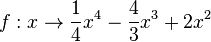
Gf hat Schnittpunkt mit der y-Achse (0;0), da f(0)=0 ist und
Schnittpunkt mit der x-Achse (0;0), da 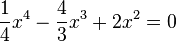
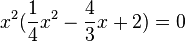
Ein Produkt ist 0, wenn ein Faktor 0 ist, also 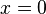 oder
oder  .
Die quadratische Gleichung hat keine Lösung, da ihre Diskriminante
.
Die quadratische Gleichung hat keine Lösung, da ihre Diskriminante 
Also ist x=0 doppelte Nullstelle.
Für die Monotonietabelle braucht man 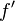 . Es ist
. Es ist 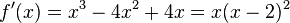
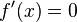 für
für  , wobei x2=2 zweifach ist.
, wobei x2=2 zweifach ist.
Gf' ist der Graph eines Polynoms 3. Grades mit Nullstelle bei x1=0 und x2=2 (doppelt), also hat man bei x2=2 keinen Vorzeichenwechsel.
Monotonietabelle:
Für x < 0 ist f'(x) < 0 und Gf ist streng monoton fallend.
Für x = 0 ist f'(0) = 0 und (0;0) ist wegen VZW -/+ ein TP.
Für 0 < x < 2 ist f'(x) > 0 und Gf ist streng monoton steigend.
Für x = 2 ist f'(2) = 0 und Gf hat dort einen Terrassenpunkt, da kein VZW.