Diskussion:M11 Skalarprodukt
Aus RSG-Wiki
Version vom 21. Januar 2021, 15:49 Uhr von Karlhaberl (Diskussion | Beiträge)
Buch S. 112 / 10
Die Vektoren 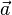 und
und  stehen senkrecht aufeinander, d.h.
stehen senkrecht aufeinander, d.h.  .
.
a) 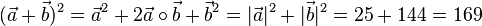
b) 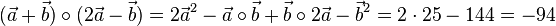
c) Eine Hommage an die binomischen Formeln!
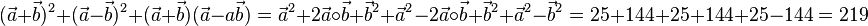
Buch S. 112 / 14
Man weiß aus der Mittelstufe, dass der Flächeninhalt eines Parallelogramms A = gh ist. D.h. fälllt man von der Spitze von  das Lot auf
das Lot auf 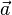 erhält man die Höhe h.
erhält man die Höhe h.
a steht für 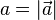 und b für
und b für 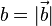 . Es ist dann
. Es ist dann  und h ist
und h ist  , also
, also 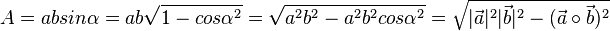 q.e.d.
q.e.d.
b) 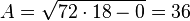 (Beachten Sie, dass
(Beachten Sie, dass 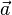 und
und  senkrecht zueinander sind.
senkrecht zueinander sind.
c) 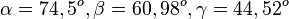 ,
, 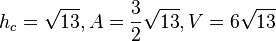
Buch S. 113 / 16
A(2;0,0), B(0;2;0), C(0;0;2) und S(0;0;0)
a) siehe Definition des Skalarprodukts
b) 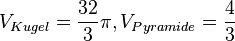 . Es ist
. Es ist