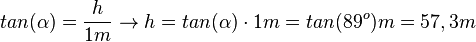M9 Aufgaben zur Trigonometrie: Unterschied zwischen den Versionen
Aus RSG-Wiki
| (10 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 7: | Zeile 7: | ||
| <math>sin(85^o)</math> || <math>cos(5^o)</math> | | <math>sin(85^o)</math> || <math>cos(5^o)</math> | ||
|- | |- | ||
| − | | <math>cos( | + | | <math>cos(10^o)</math> || <math>sin(80^o)</math> |
|- | |- | ||
| <math>cos(60^o)</math> || <math>sin(30^o)</math> | | <math>cos(60^o)</math> || <math>sin(30^o)</math> | ||
| Zeile 45: | Zeile 45: | ||
{{Lösung versteckt|1=1. <math>sin(\alpha)=\sqrt {1-(cos(\alpha))^2}</math> , <math>cos(\alpha)=\sqrt {1-(sin(\alpha))^2}</math><br> | {{Lösung versteckt|1=1. <math>sin(\alpha)=\sqrt {1-(cos(\alpha))^2}</math> , <math>cos(\alpha)=\sqrt {1-(sin(\alpha))^2}</math><br> | ||
| − | 2. a)<math>cos(\alpha)=\sqrt {1-(sin(\alpha))^2}=\sqrt {1-(0,25)^2}= | + | 2. a)<math>cos(\alpha)=\sqrt {1-(sin(\alpha))^2}=\sqrt {1-(0,25)^2}=0,968</math><br> |
<math>tan(\varphi)=\frac{sin(\varphi)}{cos(\varphi)}=\frac{0,25}{0,968}=0,258</math> | <math>tan(\varphi)=\frac{sin(\varphi)}{cos(\varphi)}=\frac{0,25}{0,968}=0,258</math> | ||
| Zeile 51: | Zeile 51: | ||
<math>tan(\varphi)=\frac{sin(\varphi)}{cos(\varphi)}=\frac{0,866}{0,5}=1,732</math> | <math>tan(\varphi)=\frac{sin(\varphi)}{cos(\varphi)}=\frac{0,866}{0,5}=1,732</math> | ||
| − | c) <math>tan(\varphi)= 1</math> bedeutet <math>\varphi = | + | c) <math>tan(\varphi)= 1</math> bedeutet <math>\varphi = 45^o</math> und <math>sin(45^o)=cos(45^o)= \frac{1}{2}\sqrt 2</math> |
| − | d) <math>cos(\varphi)=0,994, tan(\varphi)= | + | d) <math>cos(\varphi)=0,994, tan(\varphi)=0,111</math> |
e) <math>sin(\varphi)=0,694, tan(\varphi)=0,964</math> | e) <math>sin(\varphi)=0,694, tan(\varphi)=0,964</math> | ||
| − | f) <math>cos(\varphi)=0,866, tan(\varphi)= | + | f) <math>cos(\varphi)=0,866, tan(\varphi)=0,577</math> }} |
| − | {{Aufgaben-blau|3|2=Buch S. 138 / 3 a - e }} | + | {{Aufgaben-blau|3|2=Buch S. 138 / 3 a - e <br> |
| + | Buch S. 138 / 5 a, b}} | ||
| − | {{Lösung versteckt|1= | + | {{Lösung versteckt|1=138/3a) Im Dreieck sind An- und Gegenkathete zum Winkel <math>\beta</math>. Also <math>tan(\beta)=\frac{2cm}{6cm}=\frac{1}{3}</math> ergibt <math>\beta = 18,4^o</math>. |
| − | b) Zum Winkel 25<sup>o</sup> ist die Hypotenuse gegeben. Gesucht ist seine Gegenkathete. Also <math>sin(25^o)=\frac{x}{7cm} \rightarrow x = 7cm \cdot sin(25^)=3,0cm</math>. | + | b) Zum Winkel 25<sup>o</sup> ist die Hypotenuse gegeben. Gesucht ist seine Gegenkathete. Also <math>sin(25^o)=\frac{x}{7cm} \rightarrow x = 7cm \cdot sin(25^o)=3,0cm</math>. |
c) Im Dreieck sind Ankathete und Hypotenuse zum Winkel <math>\gamma</math>. Also <math>cos(\gamma)=\frac{4cm}{10cm}=0,4</math> ergibt <math>\gamma = 66,4^o</math>. | c) Im Dreieck sind Ankathete und Hypotenuse zum Winkel <math>\gamma</math>. Also <math>cos(\gamma)=\frac{4cm}{10cm}=0,4</math> ergibt <math>\gamma = 66,4^o</math>. | ||
| Zeile 80: | Zeile 81: | ||
Im rechten Dreieck ist die Gegenkathete zum 12<sup>o</sup>-Winkel gesucht und die Ankathete gegeben. Also <math>tan(12^o)=\frac{x}{7,2cm} \rightarrow x = 7,2cm \cdot tan(12^o)=1,5cm</math> | Im rechten Dreieck ist die Gegenkathete zum 12<sup>o</sup>-Winkel gesucht und die Ankathete gegeben. Also <math>tan(12^o)=\frac{x}{7,2cm} \rightarrow x = 7,2cm \cdot tan(12^o)=1,5cm</math> | ||
| − | Da es sich hier um ein Parallelogramm handelt, hätte man x auch mit dem Satz von Pythagoras berechnen können. | + | Da es sich hier um ein Parallelogramm handelt, hätte man x auch mit dem Satz von Pythagoras berechnen können. |
| − | + | --------------------------------------- | |
| − | + | 138/5a) In dem Dreieck sind a, b, und <math>\gamma</math> gegeben, also nimmt man als Überlegungsfigur das rechte Dreieck. Man sieht, dass man h<sub>b</sub> berechnen kann. Es ist <math>sin(\gamma)=\frac{h_b}{a} \rightarrow h_b=a\cdot sin(\gamma)=4cm \cdot sin(45^o)=4cm \cdot \frac{1}{2}\sqrt 2=2\sqrt 2cm\approx 2,8cm</math> <br> | |
Damit ist der Flächeninhalt <math>A = \frac{1}{2}bh_b=\frac{1}{2}\cdot 7cm \cdot 2\sqrt 2cm =7\sqrt 2 cm^2\approx 9,9cm^2</math> | Damit ist der Flächeninhalt <math>A = \frac{1}{2}bh_b=\frac{1}{2}\cdot 7cm \cdot 2\sqrt 2cm =7\sqrt 2 cm^2\approx 9,9cm^2</math> | ||
| + | |||
| + | b) Im Dreieck sind b, C und amath>\alpha</math> gegeben, also nimmt man das linke Dreieck als Überlegungsfigur. Man kann h<sub>c</sub> berechnen. Es ist <math>sin(\alpha)=\frac{h_c}{b} \rightarrow h_c = b\cdot sin(\alpha)=6cm \cdot sin(60^o)=6cm \cdot \frac{1}{2}\sqrt 3 = 3 \sqrt 3 cm \approx 5,2cm</math>.<br> | ||
| + | Der Flächeninhalt ist <math>A=\frac{1}{2}ch_c=\frac{1}{2}\cdot 4cm \cdot 3\sqrt 3cm =6\sqrt 3 cm^2\approx 10,4cm^2</math> }} | ||
| + | |||
| + | {{Aufgaben-blau|4|2=Textaufgaben<br> | ||
| + | Buch S. 139 / 10 <br> | ||
| + | Buch S. 140 /16 <br> | ||
| + | Buch S. 140 / 17 <br> | ||
| + | Buch S. 140 / 15<br> | ||
| + | Buch S. 140 / 19}} | ||
| + | |||
| + | {{Lösung versteckt|1=139/10a) In dem rechtwinkligen Dreieck sind die Hypotenuse c = 5m und der Winkel <math>\alpha=15^o</math> gegeben. | ||
| + | Es ist <math>sin(15^o)=\frac{d}{5m} \rightarrow d = 5m \cdot sin(15^o)=1,29m</math>.<br> | ||
| + | |||
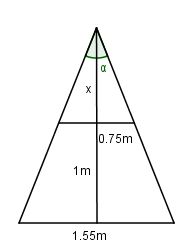
| + | b) Hier macht man sich erste eine Skizze und trägt Hilfslinien ein.<br> | ||
| + | [[Datei:139-10b.jpg]]<br> | ||
| + | In der Zeichnung kennt man keinen Winkel, aber diverse Streckenlängen, allerdings keine Längen in einem rechtwinkligen Dreieck. <br> Die Höhe auf die Basis ist in der Skizze x + 1m. Mit Hilfe des Strahlensatzes kann man x berechnen. <br> | ||
| + | <math>\frac{x}{x+1} = \frac{0,75}{1,55}</math> <br> | ||
| + | <math>1,55x = 0,75x + 0,75</math><br> | ||
| + | <math>0,8 x = 0,75</math><br> | ||
| + | <math>x=\frac{0,75}{0,8}=\frac{15}{16}=0,9375</math>.<br> | ||
| + | Also ist die Höhe h in dem gleichschenkligen Dreieck h = 1.9375m.<br> | ||
| + | Man erreicht also mit der Leiter eine Höhe von knapp 2m.<br> | ||
| + | Für diese Lösung hat man keinen Winkel, keinen Sinus, Kosinus oder Tangens gebraucht, sondern nur den Strahlensatz! | ||
| + | |||
| + | Es sollte aber auch mit sin, cos oder tan gehen. <br> | ||
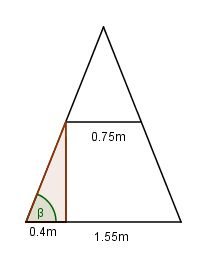
| + | [[Datei:139-10b 2.jpg]]<br> | ||
| + | In dem Dreieck links unten kennt man zum Basiswinkel <math>\beta</math> die Ankathete 0,4m und die Gegenkathete 1,0m, also ist <math>tan(\beta)=\frac{1m}{0,4m}=2,5</math> und <matsh>\beta = 68,2^o</math><br> | ||
| + | Zeichnet man nun noch die Höhe h von der Spitze des gleichschenkligen Dreiecks auf die Basis ein, dann hat man im linken rechtwinkligen Dreieck den Winkel <math>\beta</math> und seine Ankathete <math>\frac{1,55m}{s}=0,775m</math> und damit kann man die Höhe h berchnen. Es ist <math>tan(\beta)=\frac{h}{0,775m} \rightarrow h = 0,775m \cdot tan(68,2^o)=1,9376m </math> | ||
| + | |||
| + | ------------------------------------------ | ||
| + | |||
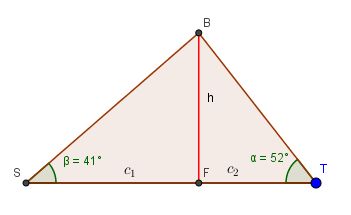
| + | 140/16 In dem Dreieck STB ist kein rechter Winkel. Man macht sich eine Skizze und trägt die Höhe von B auf die Seite [ST] ein.<br> | ||
| + | [[Datei:140-16.jpg]]<br> | ||
| + | Die Dreiecke SFB und FTB sind bei F jeweils rechtwinklig.<br> | ||
| + | Man kennt nur die beiden Winkel <math>\alpha</math> und <math>\beta</math> und keine weitere Streckenlängen in den rechtwinkligen Dreiecken. Man weiß aber, dass c<sub>1</sub> + c<sub>2</sub> = 29m ist. Also kann c<sub>1</sub> und c<sub>2</sub> verwenden. Es ist nämlich <math>tan(\alpha)=\frac{h}{c_2}, tan(\beta) = \frac{h}{c_1}</math> und damit ist <math>c_2=\frac{h}{tan(\alpha)}, c_1=\frac{h}{tan(\beta)}</math>. Setzt man dies in c<sub>1</sub> + c<sub>2</sub> = 29m ein , so erhält man <br> | ||
| + | <math>c_1 + c_2 = \frac{h}{tan(\beta)} + \frac{h}{tan(\alpha)}=h\cdot(\frac{1}{tan(\alpha)}+\frac{1}{tan(\beta)})=29m</math>.<br> | ||
| + | Nach h aufgelöst: <math> h = \frac{29m}{\frac{1}{tan(\alpha)}+\frac{1}{tan(\beta)}}=\frac{29m}{\frac{1}{tan(52^o)}+\frac{1}{tan(41^o)}}=15m</math>. <br> | ||
| + | Der Fluss ist 15m breit. | ||
| + | |||
| + | ------------------------------------------------------- | ||
| + | |||
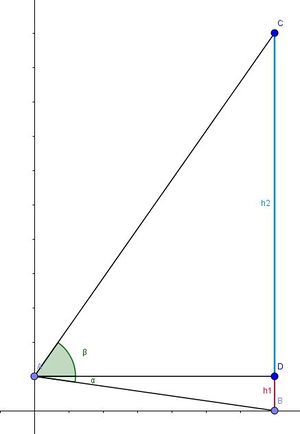
| + | 140/17 In dem Bild ist <math>\overline {AD} = 70m, \alpha = 10^o, \beta = 55^o</math>.<br> | ||
| + | [[Datei:140-17.jpg|300px]]<br> | ||
| + | Es ist <math>tan(\alpha)=\frac{h_1}{70m}, tan(\beta)=\frac{h2}{70m}</math>. <br> | ||
| + | <math>h_1=tan(\alpha)\cdot 70m = tan(10^o)\cdot 70m = 12,34m, h2=tan(\beta)\cdot 70m=tan(55^o) \cdot 70m=99,97m, h_1+h_2=112,31m\approx 110m</math> | ||
| + | |||
| + | ---------------------------------------------------------- | ||
| + | |||
| + | 140/15{[[Datei:140-15.jpg|300px]]<br> | ||
| + | Das Bild ist symmetrisch, daher reicht es auf einer Seite den Winkel zu berechnen. Im rechten Dreieck BFD ist <math>cos(\varphi)=\frac{50ft}{100ft}=\frac{1}{2} \rightarrow \varphi = 60^o</math>. | ||
| + | |||
| + | ---------------------------------------------------------- | ||
| + | |||
| + | 140/19<br> | ||
| + | [[Datei:140-19.jpg]] | ||
| + | |||
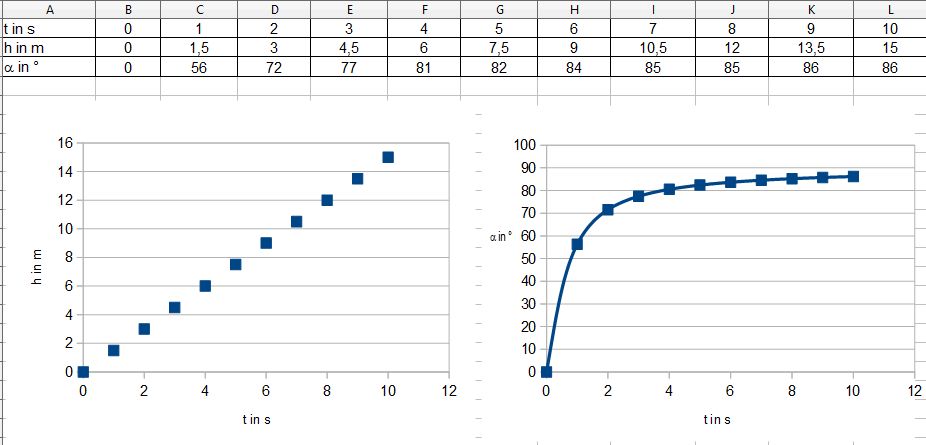
| + | c) <math>tan(\alpha)=\frac{h}{1m} \rightarrow h = tan(\alpha)\cdot 1m=tan(89^o)m=57,3m</math> }} | ||
Aktuelle Version vom 29. April 2021, 10:33 Uhr
Ordne richtig zu
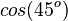
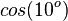
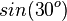
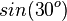
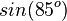
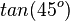
0
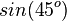
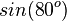 0,5
0,5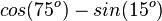
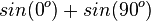
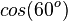

|
Merke:
 |
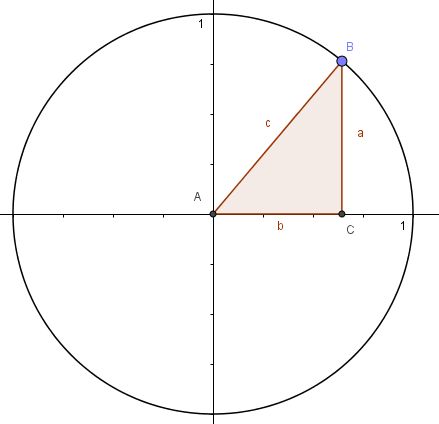
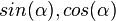 .
. und
und 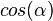 erhältst du?
erhältst du?
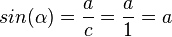
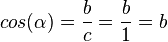
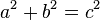

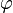 für
für 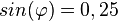 die Werte von
die Werte von 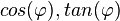 .
.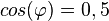 die Werte von
die Werte von 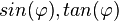 .
.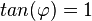 die Werte von
die Werte von 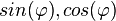 .
.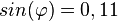 die Werte von
die Werte von 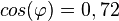 die Werte von
die Werte von 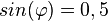 die Werte von
die Werte von  ,
, 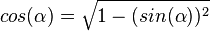

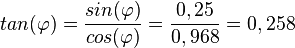


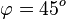 und
und 

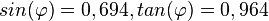

 . Also
. Also 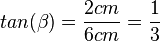 ergibt
ergibt 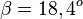 .
.
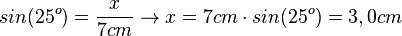 .
.
 . Also
. Also 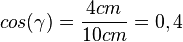 ergibt
ergibt  .
.
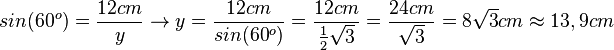
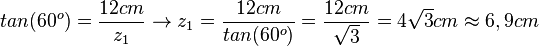 oder
oder 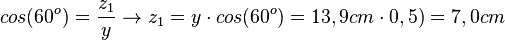 . Die unterschiedlichen Ergebnisse 6,9 cm und 7,0cm ergeben sich aus den Rundungen, mit denen man weiterrechnet. Wenn man die gnaze Anzeige des Taschenrechners nimmt und mit ihr weiterrechnet, erhält man in beiden Fällen 6,9cm.
. Die unterschiedlichen Ergebnisse 6,9 cm und 7,0cm ergeben sich aus den Rundungen, mit denen man weiterrechnet. Wenn man die gnaze Anzeige des Taschenrechners nimmt und mit ihr weiterrechnet, erhält man in beiden Fällen 6,9cm.
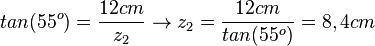 ,
, 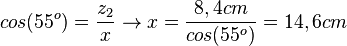

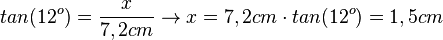
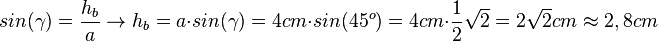
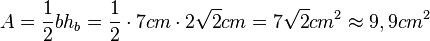
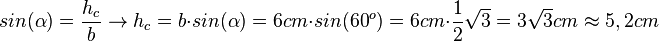 .
.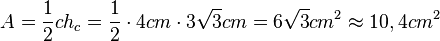
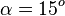 gegeben.
Es ist
gegeben.
Es ist 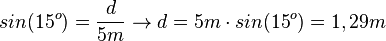 .
.
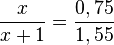

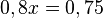
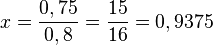 .
.
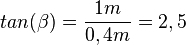 und <matsh>\beta = 68,2^o</math>
und <matsh>\beta = 68,2^o</math>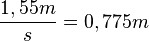 und damit kann man die Höhe h berchnen. Es ist
und damit kann man die Höhe h berchnen. Es ist 
 und
und  und damit ist
und damit ist 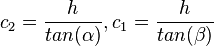 . Setzt man dies in c1 + c2 = 29m ein , so erhält man
. Setzt man dies in c1 + c2 = 29m ein , so erhält man 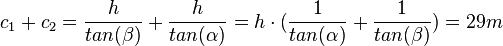 .
.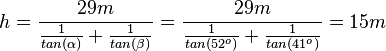 .
. 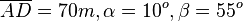 .
.
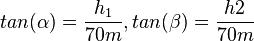 .
. 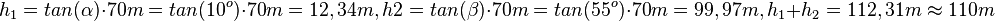

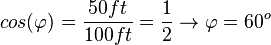 .
.