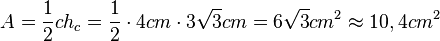M9 Aufgaben zur Trigonometrie: Unterschied zwischen den Versionen
Aus RSG-Wiki
| Zeile 59: | Zeile 59: | ||
f) <math>cos(\varphi)=0,866, tan(\varphi)=0577</math> }} | f) <math>cos(\varphi)=0,866, tan(\varphi)=0577</math> }} | ||
| − | {{Aufgaben-blau|3|2=Buch S. 138 / 3 a - e }} | + | {{Aufgaben-blau|3|2=Buch S. 138 / 3 a - e <br> |
| + | Buch S. 138 / 5 a, b}} | ||
| − | {{Lösung versteckt|1= | + | {{Lösung versteckt|1=138/3a) Im Dreieck sind An- und Gegenkathete zum Winkel <math>\beta</math>. Also <math>tan(\beta)=\frac{2cm}{6cm}=\frac{1}{3}</math> ergibt <math>\beta = 18,4^o</math>. |
b) Zum Winkel 25<sup>o</sup> ist die Hypotenuse gegeben. Gesucht ist seine Gegenkathete. Also <math>sin(25^o)=\frac{x}{7cm} \rightarrow x = 7cm \cdot sin(25^)=3,0cm</math>. | b) Zum Winkel 25<sup>o</sup> ist die Hypotenuse gegeben. Gesucht ist seine Gegenkathete. Also <math>sin(25^o)=\frac{x}{7cm} \rightarrow x = 7cm \cdot sin(25^)=3,0cm</math>. | ||
| Zeile 80: | Zeile 81: | ||
Im rechten Dreieck ist die Gegenkathete zum 12<sup>o</sup>-Winkel gesucht und die Ankathete gegeben. Also <math>tan(12^o)=\frac{x}{7,2cm} \rightarrow x = 7,2cm \cdot tan(12^o)=1,5cm</math> | Im rechten Dreieck ist die Gegenkathete zum 12<sup>o</sup>-Winkel gesucht und die Ankathete gegeben. Also <math>tan(12^o)=\frac{x}{7,2cm} \rightarrow x = 7,2cm \cdot tan(12^o)=1,5cm</math> | ||
| − | Da es sich hier um ein Parallelogramm handelt, hätte man x auch mit dem Satz von Pythagoras berechnen können. | + | Da es sich hier um ein Parallelogramm handelt, hätte man x auch mit dem Satz von Pythagoras berechnen können. |
| − | + | --------------------------------------- | |
| − | + | 138/5a) In dem Dreieck sind a, b, und <math>\gamma</math> gegeben, also nimmt man als Überlegungsfigur das rechte Dreieck. Man sieht, dass man h<sub>b</sub> berechnen kann. Es ist <math>sin(\gamma)=\frac{h_b}{a} \rightarrow h_b=a\cdot sin(\gamma)=4cm \cdot sin(45^o)=4cm \cdot \frac{1}{2}\sqrt 2=2\sqrt 2cm\approx 2,8cm</math> <br> | |
Damit ist der Flächeninhalt <math>A = \frac{1}{2}bh_b=\frac{1}{2}\cdot 7cm \cdot 2\sqrt 2cm =7\sqrt 2 cm^2\approx 9,9cm^2</math> | Damit ist der Flächeninhalt <math>A = \frac{1}{2}bh_b=\frac{1}{2}\cdot 7cm \cdot 2\sqrt 2cm =7\sqrt 2 cm^2\approx 9,9cm^2</math> | ||
| + | |||
| + | b) Im Dreieck sind b, C und amath>\alpha</math> gegeben, also nimmt man das linke Dreieck als Überlegungsfigur. Man kann h<sub>c</sub> berechnen. Es ist <math>sin(\alpha)=\frac{h_c}{b} \rightarrow h_c = b\cdot sin(\alpha)=6cm \cdot sin(60^o)=6cm \cdot \frac{1}{2}\sqrt 3 = 3 \sqrt 3 cm \approx 5,2cm</math>.<br> | ||
| + | Der Flächeninhalt ist <math>A=\frac{1}{2}ch_c=\frac{1}{2}\cdot 4cm \cdot 3\sqrt 3cm =6\sqrt 3 cm^2\approx 10,4cm^2</math> }} | ||
| + | |||
| + | {{Aufgaben-blau|4|2= }} | ||
Version vom 22. April 2021, 11:09 Uhr
Ordne richtig zu
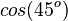
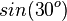
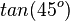
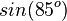
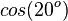
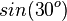
0
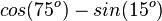
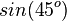 0,5
0,5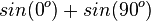
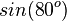
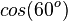

|
Merke:
 |
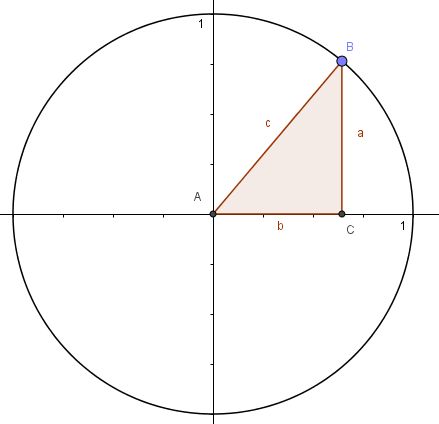
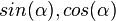 .
. und
und 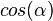 erhältst du?
erhältst du?
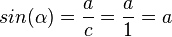
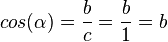
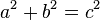

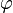 für
für 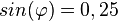 die Werte von
die Werte von 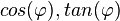 .
.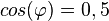 die Werte von
die Werte von 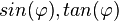 .
.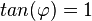 die Werte von
die Werte von 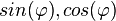 .
.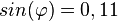 die Werte von
die Werte von 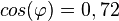 die Werte von
die Werte von 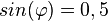 die Werte von
die Werte von  ,
, 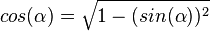
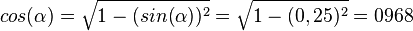
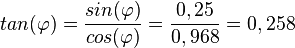


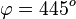 und
und 
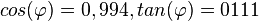
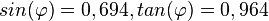
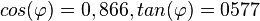
 . Also
. Also 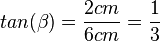 ergibt
ergibt 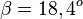 .
.
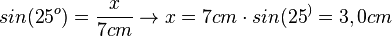 .
.
 . Also
. Also 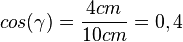 ergibt
ergibt  .
.
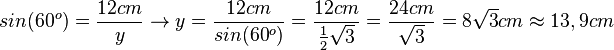
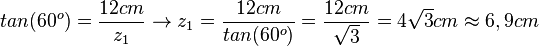 oder
oder 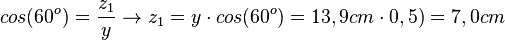 . Die unterschiedlichen Ergebnisse 6,9 cm und 7,0cm ergeben sich aus den Rundungen, mit denen man weiterrechnet. Wenn man die gnaze Anzeige des Taschenrechners nimmt und mit ihr weiterrechnet, erhält man in beiden Fällen 6,9cm.
. Die unterschiedlichen Ergebnisse 6,9 cm und 7,0cm ergeben sich aus den Rundungen, mit denen man weiterrechnet. Wenn man die gnaze Anzeige des Taschenrechners nimmt und mit ihr weiterrechnet, erhält man in beiden Fällen 6,9cm.
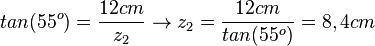 ,
, 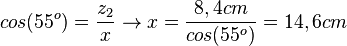

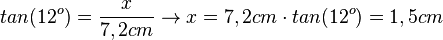
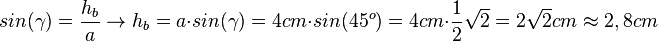
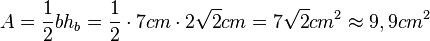
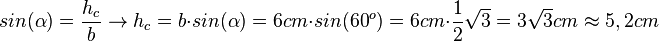 .
.