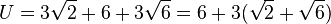M11 Betrag eines Vektors: Unterschied zwischen den Versionen
Aus RSG-Wiki
| Zeile 86: | Zeile 86: | ||
d) gleich sind keine der Vektoren | d) gleich sind keine der Vektoren | ||
| − | }} | + | 106/7a) <math>|\vec {AB}|=7</math><br> |
| + | b) <math>|\vec {AB}|=10</math><br> | ||
| + | c) <math>|\vec {AB}|=17</math><br> | ||
| + | |||
| + | 106/8 a) <math> |\vec {AB}|=\left | \left ( \begin{array}{c} -8 \\\ k-1 \\\ -1 \end{array}\right) \right| =\sqrt{64+(k-1)^2+1}=9</math> , also <math>65 + (k-1)^2=81</math> --> <math>(k-1)^2 = 16</math> dies liefert zwei Lösungen <br> | ||
| + | <math>k_1-1=-4 \rightarrow k_1=-3</math> und <math> k_2-1=4 \rightarrow k_2 = 5</math><br> | ||
| + | b) k<sub>1</sub>=3 und k<sub>2</sub>=7<br> | ||
| + | |||
| + | 106/9 Die Dreiecksseiten sind <math>c=|\vec{AB}|, a=|\vec{BC}|, b=|\vec{AC}|</math><br> | ||
| + | <math>c=|\vec{AB}|=\left | \left ( \begin{array}{c} 3 \\\ 0 \\\ -3 \end{array}\right) \right|=3\sqrt 2</math><br> | ||
| + | <math>a=|\vec{BC}|=\left | \left ( \begin{array}{c} 0 \\\ 6 \\\ 0 \end{array}\right) \right|=6</math><br> | ||
| + | <math>b=|\vec{AC}|=\left | \left ( \begin{array}{c} 3 \\\ 6 \\\ -3 \end{array}\right) \right|=3\sqrt {6}</math><br> | ||
| + | Ein Dreieck ist rechtwinklig, wenn der Satz des Pythagoras gilt: <math>c^2+a^2=18+36=54=b^2</math>, also ist das Dreieck ABC bei B rechtwinklig. <br> | ||
| + | Der Flächeninhalt ergibt sich zu <math>A=\frac{1}{2}\cdot 3\sqrt 2 \cdot 6= 9\sqrt 2 </math><br> | ||
| + | Die Umfangslänge ist <math>U=3\sqrt 2 + 6 + 3\sqrt {6}=6+3(\sqrt 2 + \sqrt 6)</math> }} | ||
Version vom 19. Januar 2021, 12:43 Uhr
|
Merke:
Der Betrag Die Entfernung zweier Punkte P und Q ist der Betrag des Vektors 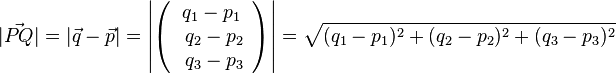 |
|
Merke:
Ein Vektor mit dem Betrag 1 heißt Einheitsvektor. |
|
Merke:
Alle Punkte X(x1,x2,x3) im Raum, die von einem Punkt M(m1,m2,m3) die gleiche Entfernung r haben bilden die Oberfläche einer Kugel K. Für die Punkte X gilt: Die Gleichung |

 des Vektors
des Vektors 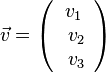 ist
ist 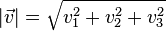 .
.
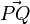 .
.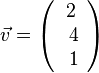 ?
?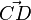 für C(-5;3,-1) und D(2;4;1).
für C(-5;3,-1) und D(2;4;1).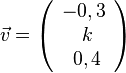 den Betrag 1?
den Betrag 1?

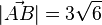
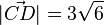
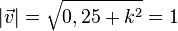 , also 0,25 + k² =1 liefert
, also 0,25 + k² =1 liefert 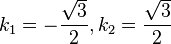
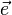 bezeichnet.
bezeichnet. 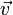 wird mit
wird mit 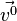 bezeichnet. Es ist
bezeichnet. Es ist 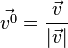
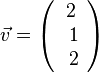 .
.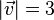 .
.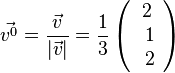

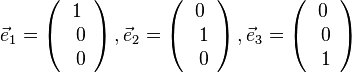
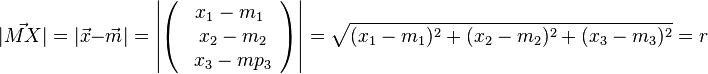
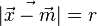 ist die Vektorgleichung,
ist die Vektorgleichung, 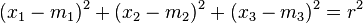 die Koordinatengleichung einer Kugel.
die Koordinatengleichung einer Kugel.
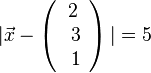 und
und 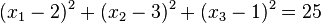
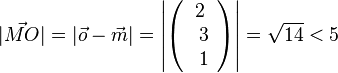 , also liegt O innerhalb der Kugel.
, also liegt O innerhalb der Kugel.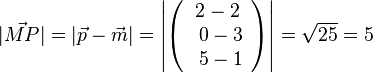 , also liegt P auf der Kugel.
, also liegt P auf der Kugel.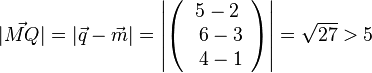 , also liegt Q außerhalb der Kugel.
, also liegt Q außerhalb der Kugel.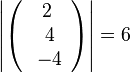


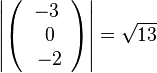
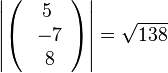
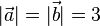 , also
, also 

 liefert
liefert  , also
, also 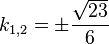
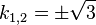
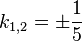
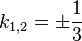
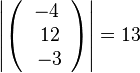 , also
, also 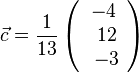
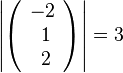 , also
, also 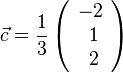
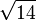 haben die Vektoren
haben die Vektoren 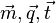 , den Betrag 2 haben die Vektoren
, den Betrag 2 haben die Vektoren 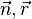 , den Betrag
, den Betrag 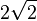 haben die Vektoren
haben die Vektoren 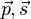
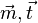 und
und 
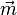 und
und 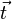 , sowie
, sowie 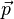 und
und 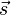 .
.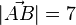
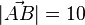
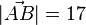
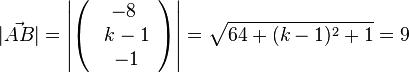 , also
, also 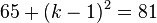 -->
--> 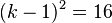 dies liefert zwei Lösungen
dies liefert zwei Lösungen  und
und 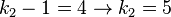
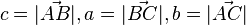
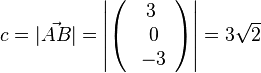
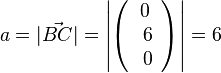

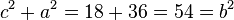 , also ist das Dreieck ABC bei B rechtwinklig.
, also ist das Dreieck ABC bei B rechtwinklig.