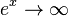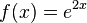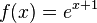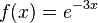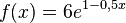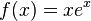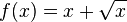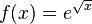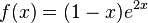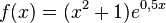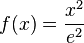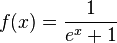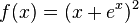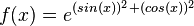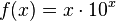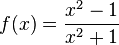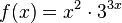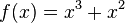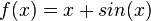M11 Ableitung der Exponentialfunktionen: Unterschied zwischen den Versionen
| (8 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
| − | + | {{Aufgaben-blau|Zur Wiederholung|2= 1. [[M10_Die_Exponentialfunktion|Die Exponentialfunktion]] | |
| − | + | ||
| − | 1. [[M10_Die_Exponentialfunktion|Die Exponentialfunktion]] | + | |
2. [[M10_Eigenschaften_der_Exponentialfunktion|Eigenschaften der Exponentialfunktion]] | 2. [[M10_Eigenschaften_der_Exponentialfunktion|Eigenschaften der Exponentialfunktion]] | ||
| − | 3. [[M10_Verschieben_und_Spiegeln_der_Exponentialkurven|Verschieben und Spiegeln der Exponentialfunktion]] | + | 3. [[M10_Verschieben_und_Spiegeln_der_Exponentialkurven|Verschieben und Spiegeln der Exponentialfunktion]] }} |
{{Merksatz|MERK=Die Funktion <math>f: R \rightarrow R, f(x) = b\cdot a^x</math> (b ∈ R+\{0}, a ∈ R<sup>+</sup>) heißt '''Exponentialfunktion zur Basis a'''. | {{Merksatz|MERK=Die Funktion <math>f: R \rightarrow R, f(x) = b\cdot a^x</math> (b ∈ R+\{0}, a ∈ R<sup>+</sup>) heißt '''Exponentialfunktion zur Basis a'''. | ||
| Zeile 12: | Zeile 10: | ||
Für die Ableitung der Exponentialfunktion zur Basis a geht man auf die Definition zurück:<br> | Für die Ableitung der Exponentialfunktion zur Basis a geht man auf die Definition zurück:<br> | ||
| − | <math>f'(x) = \lim_{h\to 0}\frac{b^{x+h}-b^x}{h}=\lim_{h\to 0} b^x \cdot \frac{b^h -1}{h}=b^x \cdot \lim_{h\to 0}\frac{b^h - 1}{h}</math> | + | <math>f'(x) = \lim_{h\to 0}\frac{b^{x+h}-b^x}{h}=\lim_{h\to 0} b^x \cdot \frac{b^h -1}{h}=b^x \cdot \lim_{h\to 0}\frac{b^h - 1}{h}</math>.<br> |
| + | Löst man die Gleichung <math>\lim_{h\to 0} \frac{b^h - 1}{h}=1</math> nach b auf, so erhält man <math>b = \lim_{h \to 0} (1+h)^{\frac{1}{h}}</math>. Wenn <math> h \to 0</math> ist, dann ist <math> n = \frac{1}{h}\to \infty</math> und man kann mittels einer Tabellenkalkulation den Grenzwert <math>\lim_{n\to \infty} \left ( 1 + \frac{1}{n} \right )^n = 2, 718 281 828 459 045 235 360 287 471 352 ...</math> berechnen.<br> Dieser Grenzwert wird als Eulersche Zahl e bezeichnet. Es ist e = 2, 718 281 828 459 045 235 360 287 471 352 ... . | ||
| + | |||
| + | <center>{{#ev:youtube |4zKwqdfuRz4|350}}</center> | ||
| Zeile 22: | Zeile 23: | ||
Jede Funktion <math>F: x \to e^x + C</math> ist Stammfunktion von <math>f</math>. | Jede Funktion <math>F: x \to e^x + C</math> ist Stammfunktion von <math>f</math>. | ||
| + | |||
| + | Die Gleichung <math>e^x = a</math> hat die Lösung <math>x = ln(a)</math>. Dabei ist <math>ln</math> der Logarithmus zur Basis e und heißt '''natürlicher Logarithmus'''. | ||
Mit <math>a^x = e^{x \cdot ln(a)}</math> und der Kettenregel erhält man <br> | Mit <math>a^x = e^{x \cdot ln(a)}</math> und der Kettenregel erhält man <br> | ||
<center><math> (a^x)' = ln(a) \cdot a^x</math>.</center> }} | <center><math> (a^x)' = ln(a) \cdot a^x</math>.</center> }} | ||
| + | |||
| + | {{Merke|1=Eigenschaften der e-Funktion (natürliche Exponentialfunktion) <math>f:x \rightarrow e^x</math> [[Datei:e-funktion.jpg|thumb|350px]] | ||
| + | |||
| + | * D = R, W = R<sup>+</sup><br> | ||
| + | |||
| + | * e<sup>x</sup> > 0 | ||
| + | |||
| + | * Der Graph geht durch den Punkt (0;1)<br> | ||
| + | |||
| + | * f(1) = e | ||
| + | |||
| + | * Der Graph der e-Funktion ist streng montoton steigend. | ||
| + | |||
| + | * Die negative x-Achse ist Asymptote. | ||
| + | |||
| + | * Für <math>x \to \infty</math> ist <math>e^x \to \infty</math>}} | ||
| + | |||
| + | |||
{{Aufgaben-blau|1|2=Bestimmen Sie die erste Ableitung<br> | {{Aufgaben-blau|1|2=Bestimmen Sie die erste Ableitung<br> | ||
| Zeile 80: | Zeile 101: | ||
x) <math>f(x) = |x-1|</math> <br> | x) <math>f(x) = |x-1|</math> <br> | ||
Bei x = 1 hat die Funktion einen Knick. Für x < 1 ist f(x) = -x + 1 und f'(x) = -1; für x < 1 ist f(x) = x -1 und f'(x) = 1.}} | Bei x = 1 hat die Funktion einen Knick. Für x < 1 ist f(x) = -x + 1 und f'(x) = -1; für x < 1 ist f(x) = x -1 und f'(x) = 1.}} | ||
| + | |||
| + | {{Aufgaben-blau|2|2=Ordnen Sie dem Term f(x) eine passende Stammfunktion F(x) zu bzw. umkehrt der Stammfunktion F(x) eine passende Funktion f(x). }} | ||
| + | |||
| + | <div class="zuordnungs-quiz"> | ||
| + | {| | ||
| + | | <math>f(x) = 2e^{2x} - 2e^{-2x}</math> || <math>F(x) = (e^x + e^{-x})^2</math> | ||
| + | |- | ||
| + | | <math>f(x) = 1-\frac{1}{e^x}</math> || <math>F(x) = x + e^{-x}</math> | ||
| + | |- | ||
| + | | <math> f(x) = e^2 \cdot e^x</math> || <math>F(x) = e^{x+2}</math> | ||
| + | |- | ||
| + | | <math>f(x) = 3x^2 + e^{3x}</math> || <math>F(x) = x^3 + \frac{1}{3}e^{3x}</math> | ||
| + | |} | ||
| + | </div> | ||
| + | |||
| + | {{Aufgaben-blau|3|2=Geben Sie jeweils eine Stammfunktion F der Funktion f an<br> | ||
| + | a) f(x) = e<sup>x</sup> + 1<br> | ||
| + | b) f(x) = e<sup>-x</sup><br> | ||
| + | c) f(x) = 0,5(e<sup>x</sup> + e<sup>-x</sup>)<br> | ||
| + | d) f(x) = x + 2 + e<sup>x+2</sup><br> | ||
| + | e) f(x) = e<sup>1+x</sup><br> | ||
| + | f) f(x) = e<sup>0,5x</sup> }} | ||
| + | |||
| + | {{Lösung versteckt|1=a) F(x) = e<sup>x</sup> + x + C<br> | ||
| + | b) F(x) = -e<sup>-x</sup> + C<br> | ||
| + | c) F(x) = 0,5(e<sup>x</sup> - e<sup>-x</sup>) + C<br> | ||
| + | d) F(x) = 0,5x<sup>2</sup> + 2x + e<sup>x+2</sup> + C<br> | ||
| + | e) F(x) = e<sup>1+x</sup> + C<br> | ||
| + | f) F(x) = 2e<sup>0,5x</sup> + C }} | ||
| + | |||
| + | <center>{{#ev:youtube |io11cbrTTNg|350}}</center> | ||
Aktuelle Version vom 19. März 2021, 14:59 Uhr
|
Merke:
Die Funktion 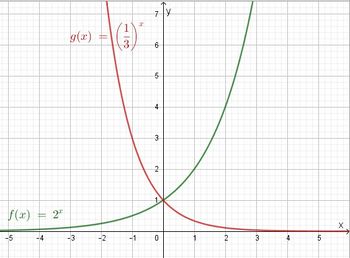 Der Graph ist eine Exponentialkurve. |
Für die Ableitung der Exponentialfunktion zur Basis a geht man auf die Definition zurück:
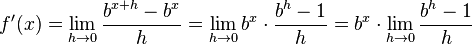 .
.
Löst man die Gleichung 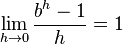 nach b auf, so erhält man
nach b auf, so erhält man 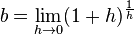 . Wenn
. Wenn 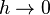 ist, dann ist
ist, dann ist  und man kann mittels einer Tabellenkalkulation den Grenzwert
und man kann mittels einer Tabellenkalkulation den Grenzwert  berechnen.
berechnen.
Dieser Grenzwert wird als Eulersche Zahl e bezeichnet. Es ist e = 2, 718 281 828 459 045 235 360 287 471 352 ... .
|
Merke:
Die Eulersche Zahl e ist definiert durch den Grenzwert Dies hat zur Folge, dass die Exponentialfunktion zur Basis e 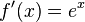 oder oder 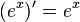 hat. Jede Funktion Die Gleichung
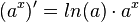 . . |
Eigenschaften der e-Funktion (natürliche Exponentialfunktion) 
|
Beachten Sie die Ableitungsregeln, insbesondere die Kettenregel.
a) 
b) 
c) 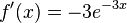
d) 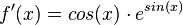
e) 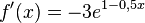
f) 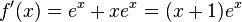
g) 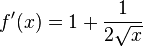
h) 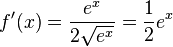
Dies hätte man auch erhalten, wenn man 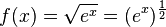 ableitet.
ableitet.
i) 
j) 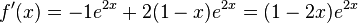
k) 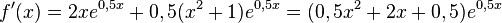
l)  - Beachte e2 ist eine Zahl und hier steht keine Exponentialfunktion!
- Beachte e2 ist eine Zahl und hier steht keine Exponentialfunktion!
m) 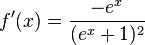
n) 
o) 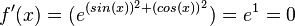
p) 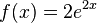
q) 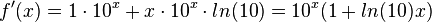
r) 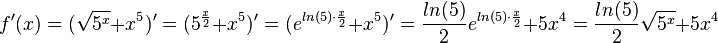
s) 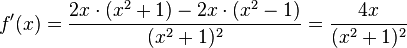
t) 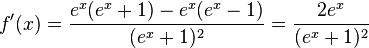
u) 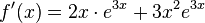
v) 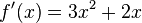
w) 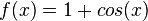
x) 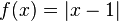
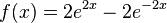 |
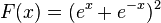
|
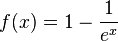 |
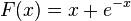
|
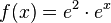 |
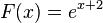
|
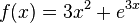 |
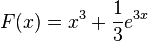
|
a) F(x) = ex + x + C
b) F(x) = -e-x + C
c) F(x) = 0,5(ex - e-x) + C
d) F(x) = 0,5x2 + 2x + ex+2 + C
e) F(x) = e1+x + C

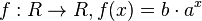 (b ∈ R+\{0}, a ∈ R+) heißt Exponentialfunktion zur Basis a.
(b ∈ R+\{0}, a ∈ R+) heißt Exponentialfunktion zur Basis a.
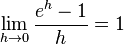 .
.
 mit D = R und W = R+ die Ableitung
mit D = R und W = R+ die Ableitung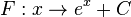 ist Stammfunktion von
ist Stammfunktion von 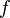 .
.
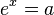 hat die Lösung
hat die Lösung 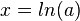 . Dabei ist
. Dabei ist 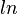 der Logarithmus zur Basis e und heißt natürlicher Logarithmus.
der Logarithmus zur Basis e und heißt natürlicher Logarithmus.
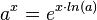 und der Kettenregel erhält man
und der Kettenregel erhält man 
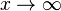 ist
ist